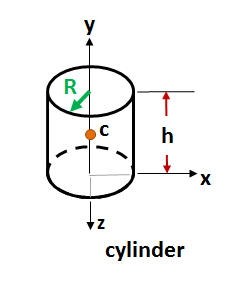
This equation computes the mass moment of inertia of a solid cylinder rotating about the z axis as shown in the diagram.
Inputs
The inputs to this calculation are:
- m= mass of the entire body
- h = Height of a Cylinder
- R = Radius of the cylinder.
Description
The Mass Moment of Inertia of a solid measures the solid's ability to resist changes in rotational speed about a specific axis. The larger the mass moment of inertia the smaller the angular acceleration about that axis for a given applied torque.
This equation computes the mass moment of inertia for a solid cylinder rotating about the z-axis as defined in the picture. The mass moment of inertia in this example is the same for rotations about both the x-axis and the z-axis due to symmetry. 1
Mass Moment of Inertia = `m ((3*r^2) + (4*h^2)`)/12
author: Carol
The mass moment of inertia varies, depends on the reference axis. And thus is often specified with two subscripts to clarify the specified axis. This helps to provide unique definition of rotational axis in three-dimensional motion where rotation can occur about multiple axes.
See also
Cylinder (solid) - Radius of Gyration about x-axis
Cylinder (solid) - Radius of Gyration about y-axis
- ^ Fundamentals of Engineering. 8th edition, 2nd Revision. National Council of Examiners for Engineering and Surveying (NCEES) - 2001. ISBN 978-1-932613-59-9. pg 61