Processing...

$T_L = ( F * d_m ) / 2*( "l" + pi * mu * d_m ) / (pi* d_m - mu * "l" )+( F * mu_c * d_c )/2$

Enter a value for all fields

This equation computes the torque, $T_R$ , required to raise a load using a Square Thread Power Screw assembly.

$T_R= F*d_m/2((l+pi*mu*d_m)/ (pi*d_m- mu*l)) + (F*u_c*d_c)/2$

where

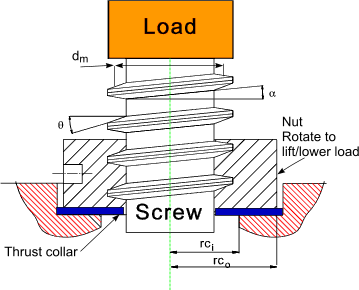
$d_m$ = mean thread diameter
$d_c$ = mean collar diameter
l = lead
F = load
$mu$ = coefficient of friction for thread
$mu_c$ = coefficient of friction for collar

/attachments/385f4b4d-c3bf-11e3-b7aa-bc764e2038f2/SquareThreadPowerScrewsTougueRequiredtoRaise-illustration.png

Notes

Square Thread Power Screws(Tougue Required to Raise)

T_R= Fd_m/2((l+πud_m)/ (πd_m- ul)) + (Fu_c*d_c)/2

where:-

d_c = Mean collar diameter

d_m = Mean Thread diameter

l =Lead

F = Load

u = coefficient of friction

u_c = coefficient of friction for the collar

This equation accounts for the force required to overcome friction between the screw and the nut in addition to the force required just to move the load. If the screw or the nut bears against a stationary surface while rotating, there will be an additional friction torque developed at that surface. For this reason, many jacks and similar devices incorporate antifriction bearings at such points.
The coefficient of friction depends on the materials used and the manner of lubricating the screw. For well-lubricated steel screws acting in steel nuts, = 0.15 should be conservative.

An important factor in the analysis for torque is the angle of inclination of the plane. In a screw thread, the angle of inclination is referred to as the lead angle, λ. It is the angle between the tangent to the helix of the thread and the plane transverse to the axis of the screw.
DL⋅π=λt
where = circumference of the pitch line of the screw pD⋅π
Then if the rotation of the screw tends to raise the load (move it up the incline), the friction force opposes the motion and acts down the plane.