20.3 Interference effects by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.

20.3 Interference effects

If you look at the front of a pair of high-quality binoculars, you will notice a greenish-blue coating on the lenses. This is advertised as a coating to prevent reflection. Now reflection is clearly undesirable --- we want the light to go in the binoculars --- but so far I've described reflection as an unalterable fact of nature, depending only on the properties of the two wave media. The coating can't change the speed of light in air or in glass, so how can it work? The key is that the coating itself is a wave medium. In other words, we have a three-layer sandwich of materials: air, coating, and glass. We will analyze the way the coating works, not because optical coatings are an important part of your education but because it provides a good example of the general phenomenon of wave interference effects.

There are two different interfaces between media: an air-coating boundary and a coating-glass boundary. Partial reflection and partial transmission will occur at each boundary. For ease of visualization let's start by considering an equivalent system consisting of three dissimilar pieces of string tied together, and a wave pattern consisting initially of a single pulse. Figure n/1 shows the incident pulse moving through the heavy rope, in which its velocity is low. When it encounters the lighter-weight rope in the middle, a faster medium, it is partially 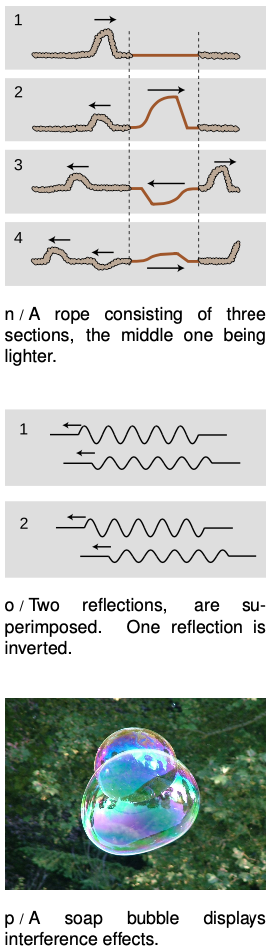reflected and partially transmitted. (The transmitted pulse is bigger, but nevertheless has only part of the original energy.) The pulse transmitted by the first interface is then partially reflected and partially transmitted by the second boundary, 3. In figure 4, two pulses are on the way back out to the left, and a single pulse is heading off to the right. (There is still a weak pulse caught between the two boundaries, and this will rattle back and forth, rapidly getting too weak to detect as it leaks energy to the outside with each partial reflection.)

Note how, of the two reflected pulses in 4, one is inverted and one uninverted. One underwent reflection at the first boundary (a reflection back into a slower medium is uninverted), but the other was reflected at the second boundary (reflection back into a faster medium is inverted).

Now let's imagine what would have happened if the incoming wave pattern had been a long sinusoidal wave train instead of a single pulse. The first two waves to reemerge on the left could be in phase, o/1, or out of phase, 2, or anywhere in between. The amount of lag between them depends entirely on the width of the middle segment of string. If we choose the width of the middle string segment correctly, then we can arrange for destructive interference to occur, 2, with cancellation resulting in a very weak reflected wave.

This whole analysis applies directly to our original case of optical coatings. Visible light from most sources does consist of a stream of short sinusoidal wave-trains such as the ones drawn above. The only real difference between the waves-on-a-rope example and the case of an optical coating is that the first and third media are air and glass, in which light does not have the same speed. However, the general result is the same as long as the air and the glass have light-wave speeds that are either both greater than the coating's or both less than the coating's.

The business of optical coatings turns out to be a very arcane one, with a plethora of trade secrets and “black magic” techniques handed down from master to apprentice. Nevertheless, the ideas you have learned about waves in general are sufficient to allow you to come to some definite conclusions without any further technical knowledge. The self-check and discussion questions will direct you along these lines of thought.

The example of an optical coating was typical of a wide variety of wave interference effects. With a little guidance, you are now ready to figure out for yourself other examples such as the rainbow pattern made by a compact disc, a layer of oil on a puddle, or a soap bubble.

self-check:

1. Color corresponds to wavelength of light waves. Is it possible to choose a thickness for an optical coating that will produce destructive interference for all colors of light?

2. How can you explain the rainbow colors on the soap bubble in figure p?

(answer in the back of the PDF version of the book)

Discussion Questions

A   Is it possible to get complete destructive interference in an optical coating, at least for light of one specific wavelength?

B   Sunlight consists of sinusoidal wave-trains containing on the order of a hundred cycles back-to-back, for a length of something like a tenth of a millimeter. What happens if you try to make an optical coating thicker than this?

C   Suppose you take two microscope slides and lay one on top of the other so that one of its edges is resting on the corresponding edge of the bottom one. If you insert a sliver of paper or a hair at the opposite end, a wedge-shaped layer of air will exist in the middle, with a thickness that changes gradually from one end to the other. What would you expect to see if the slides were illuminated from above by light of a single color? How would this change if you gradually lifted the lower edge of the top slide until the two slides were finally parallel?

D   An observation like the one described in discussion question wave theory of light! If Newton didn't know about inverting and noninverting reflections, what would have seemed inexplicable to him about the region where the air layer had zero or nearly zero thickness?

20.3 Interference effects by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.