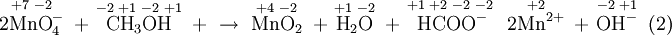CHM1 20 Balancing Redox Rxns Collection

Some redox equations may be balanced using the methods developed in Balancing Chemical Equations, but most are rather difficult to handle. Therefore it is useful to have some rules, albeit somewhat arbitrary ones, to help find appropriate coefficients. These rules depend on whether the reaction occurs in acidic or basic solution. In either situation we must make sure that the number of electrons accepted by the oxidizing agent exactly equals the number of electrons donated by the reducing agent.

In acid solution       We shall apply the rules to the equation

The changes in oxidation numbers verify that it is a redox equation, and the presence of H₃O⁺ indicates that it occurs in acidic solution. The rules are

1 Write unbalanced half-equations for the oxidation of the reducing agent and for the reduction of the oxidizing agent.

Oxidation:         O₂ ? SO₄^2–

Reduction:      IO₃^– ? I₂

2 Balance the element reduced or oxidized in each half-equation.

Oxidation:        SO₂ ? SO₄^2–...S already balanced

Reduction:      2IO₃^– ? I₂

3 Balance oxygen atoms by adding water (solvent) molecules.

Oxidation:      SO₂ + 2H₂O ? SO₄^2–

Reduction:                 IO₃^– ? I₂ + 6H₂O

4 Balance hydrogen atoms by adding hydrogen ions (available from the acidic solution).

Oxidation:      SO₂ + 2H₂O ? SO₄^2– + 2H⁺

Reduction:      12H⁺ + IO₃^– ? I₂ + 6H₂O

5 Balance electrical charges by adding electrons.

Oxidation:                  SO₂ + 2H₂O ? SO₄^2– + 2H⁺ + 2e^–

(The total charge on the left side was 0, but on the right it was – 2 + 4 = +2. Therefore 2e^– were needed on the right.)

Reduction:      10e^– + 12H⁺ + 2IO₃^– ? I₂ + 6H₂O

(The total charge on the left was 12 - 2 = +10, but on the right it was 0. Therefore 10e^– were needed on the left.)

6 Use oxidation numbers to check that the number of electrons is correct.

Oxidation: The oxidation number of electrons increases from +4 to +6, corresponding to a loss of 2e^–.

Reduction: The oxidation number of I falls from +5 to 0, corresponding to a gain of 5e^– for each I. Since there are 2 I atoms, 10e^– must be added.

7 Adjust both half-equations so that the number of electrons donated by the reducing agent equals the number of electrons accepted by the oxidizing agent. Since only 2 electrons are donated in the oxidation half-equation, while 10 are required by the reduction, the oxidation must occur 5 times for each reduction. That is, both sides of the oxidation half-equation must be multiplied by 5:

Oxidation:               5SO₂ + 10H₂O ? 5SO₄^2– + 20H⁺ + 10e^–

Reduction:      10e^– + 12H⁺ + 2IO₃^– ? I₂ + 6H₂O

8 Sum the half-equations. The net equation which result is

        5SO₂ + 4H₂O + 2IO₃^– ? 5SO₄^2– + 8H⁺ + I₂

Note that when the half-equations were summed, the number of electrons was the same on both sides, and so no free electrons (which could not exist in aqueous solution) appear in the final result. It also would be more accurate to write H₃O⁺ instead of H⁺ for the hydronium ion. This can be done by adding 8H₂O to both sides of the equation:

      5SO₂ + 12H₂O + 2IO₃^– ? 5SO₄^2– + 8 H₃O⁺ + I₂

(On the right, the 8H₂O molecules are protonated to 8H₃O⁺. It is also a good idea at this point to check that all atoms, as well as the electrical charges, balance.

In basic solution      Potassium permanganate KMnO₄, can be used to oxidize alcohols to carboxylic acids. An example is

Since OH^– is produced, the reaction occurs in basic solution. It clearly involves redox.

1 Write unbalanced equations for the oxidation of the reducing agent and the reduction of the oxidizing agent (same as for acid solution).

Oxidation:      CH₃OH ? HCOO^–

Reduction:      MnO₄^– ? MnO₂

2 Balance the element reduced or oxidized in each half-equation (same as for acid solution). (Both C and Mn are already balanced.)

3 Balance oxygen atoms by adding hydroxide ions (available from the basic solution).

Oxidation:     CH₃OH + OH^– ? HCOO^–

Reduction:               MnO₄^– ? MnO₂ + 2OH^–

4 To the side of each half-equation which lacks hydrogen, add one water molecule for each hydrogen needed. Add an equal number of hydroxide ions to the opposite side.

Oxidation:      CH₃OH + 5OH^– ? HCOO^– + 4H₂O

(Four hydrogens were needed on the right, and so 4 water molecules were added on the right and 4 hydroxide ions on the left.)

Reduction:      MnO₄^– + 2H₂O ? MnO₂ + 4OH^–

(Two hydrogens were needed on the left, and so 2 water molecules were added on the left and 2 hydroxide ions were added on the right. Note that the added hydroxide ions are to maintain the balance of oxygen atoms.)

5 Balance electrical charges by adding electrons (same as for acid solution).

Oxidation:               CH₃OH + 5OH^– ? HCOO^– + 4H₂O + 4e^–

(The total charge on the left was -5, but on the right it was -1, and so 4e^– were added on the right.)

Reduction:      MnO₄^– + 2H₂O + 3e^– ? MnO₂ + 4OH^–

(The total charge on the left was -1, but on the right it was -4, and so 3e^– were added on the left.)

6 Use oxidation numbers to check that the number of electrons is correct (same as for acid solution).

Oxidation: C goes from -2 to +2, corresponding to a loss of 4e^–.

Reduction: Mn goes from +7 to +4, corresponding to a gain of 3e^–.

7 Adjust both half-equations so that the number of electrons donated by the reducing agent equals the number of electrons accepted by the oxidizing agent (same as for acid solution). Multiplying the oxidation half-equation by 3 and the reduction half-equation by 4 adjusts each so it involves 12e^–.

Oxidation:               3CH₃OH + 15OH^– ? 3HCOO^– + 12H₂O + 12e^–

Reduction:      4MnO₄^– + 8H₂O + 12e^– ? 4MnO₂ + 16OH^–

8 Sum the half-equations (same as for acid solution). The net equation which results is

      3CH₃OH +4MnO₄^– ? 3HCOO^– + 4MnO₂ + OH^–

Again, it is worthwhile to check that all atoms and charges balance.

The rules for balancing redox equations involve adding H⁺, H₂O, and OH^– to one side or the other of the half-equations. Since these species are present in the solution, they may participate as reactants or products, but usually there is no experiment which can tell whether they do participate. However, the balanced equation derived from our rules does indicate just what role H⁺, H₂O, or OH^– play in a given redox process.