CHM1 21 Molecular Orbital Theory Collection

The Valence Bond Theory and Lewis Structures  explain simple models by treating electrons either as bonding pairs located between two nuclei, or as lone pairs associated with a single nucleus. That is, in the Valence Bond Theory electrons are said to occupy quantum-mechanical orbitals calculated for the individual atoms (solutions for Schrodinger's equation are the hybridized orbitals). While the Valence Bond Theory and Lewis Structures are sufficient for simple models, the Molecular Orbital Theory provides answers to more complex questions.The Molecular Orbital Theory, initially developed by Robert S. Mullikan, incorporates the wave like characteristics of electrons in describing bonding behavior. In Molecular Orbital Theory, the bonding between atoms is described as a combination of their atomic orbitals to form molecular orbitals (MO's) so that electrons in them belong to the molecule as a whole.

Valence bond theories and molecular orbital theories are alternate descriptions of chemical bonding that have strengths and weaknesses. Valence bond theory lends itself well to visualization. Molecular orbital theory gives better descriptions of electron cloud distributions, bond energies, and magnetic properties, but its results are not easy to visualize.

In the Molecular Orbital Theory, the electrons are delocalized. Electrons are considered delocalized when they are not assigned to a particular atom or bond (as in the case with Lewis Structures). Instead, the electrons are “smeared out” across the whole molecule. The Molecular Orbital Theory allows one to predict the distribution of electrons in a molecule which in turn can help predict molecular properties such as shape, magnetism, and Bond Order.

Introduction

Atoms form bonds by sharing electrons. Atoms can share two, four, or six electrons, forming single, double, and triple bonds respectively. Although it is impossible to determine the exact position of an electron, it is possible to calculate the probability that one will find the electron at any point around the nucleus using the Schrödinger Equation . This equation can help predict and determine the energy and spatial distribution of the electron, as well as the shape of each orbital. Figure 1 below shows the first five solutions to the equation in a three dimensional space.  

Figure 1: Atomic Orbitals

For a description of Figure 1, click the figure.

Principles of Molecular Orbital Theory

In molecules, atomic orbitals (shown by examples in Figure 1) combine to form molecular orbitals which surround the whole molecule (unlike Valence Bond Orbitals which are associated with a single atom). Similar to atomic orbitals, molecular orbitals are wave functions giving the probability of finding an electron in certain regions of a molecule. Each molecular orbital can only have 2 electrons, each with an opposite spin.  For example, if we take the 1s electron wave of one H atom and combine with the 1s electron wave of another. The electrons can overlap so that we have constructive interference between the two waves and is referred to as positive overlap. This results in a bigger electron wave (and hence more electron density) between the two atomic nuclei. This attracts the positively charged nuclei together, forming a bond. A molecular orbital formed as a result of positive overlap is called a bonding MO.

It is also possible to combine two electron waves so that destructive interference of the waves occurs between the atomic nuclei. This situation is referred to as negative overlap, and it decreases the probability of finding an electron between the nuclei. In the case of two H atoms this results in a planar node of zero electron density halfway between the nuclei. Without a buildup of negative charge between them, the nuclei repel each other and no chemical bond is possible. A molecular orbital formed as a result of negative overlap is called an antibonding MO. For further discussion of constructive vs. destructive waves, watch THIS.

To recap, in a bonding molecular orbital, the electron density is high between the two atoms, where it stabilizes the arrangement by attracting both nuclei. By contrast, an antibonding orbital has a region of zero electron density called a node between the nuclei. This allows the nuclei to repel one another more strongly, which makes the arrangement less stable. Electrons are more stable (have lower energy) in bonding molecular orbitals than in individual atoms. Placing electrons in antibonding orbitals requires an increase in their energy, which makes them less stable than in individual atoms.

Figure 2 show the molecular orbital diagram for the combination of the 1s atomic orbitals (AOs) on two identical atoms to form two new molecular orbitals (MOs). The resulting MOs include one bonding orbital (resulting from the constructive overlap of 1s wave functions) and one antibonding orbital (resulting from the destructive overlap of 1s wave functions). 

Figure 2: Molecular Orbitals of H₂

For a description of Figure 1, click the figure.
 The following are the main principles of the Molecular Orbital Theory, some of which have been described above, and other which will be described in greater detail later in the chapter.

	Principle	Details/Examples
1	Total number of molecular orbits is equal to the total number of atomic orbitals from combining atoms (found from the electron configuration and quantum numbers).	The molecule H2 is composed of two H atoms. Both H atoms have a 1s orbital, so when bonded together, there are two molecular orbitals (1 bonding and 1 antibonding).
2	When two atomic orbitals are combined you will have two resulting MOs: 1. Bonding molecular orbitals have less energy than the constituent atomic orbitals before bonding. 2. Antibonding molecular orbitals have more energy than the constituent atomic orbitals before bonding.	Bonding molecular orbitals help stabilize a system of atoms since less energy is associated with bonded atoms as opposed to a system of unbound atoms. Likewise, antibonding molecular orbitals cause a system to be unstabilized since more energy is associated with bonded atoms than that of a system of unbound atoms.
3	Following both the Pauli exclusion principle and Hund's rule, electrons fill in orbitals of increasing energy.	Electrons fill orbitals with the lowest energy first. No more than 2 electrons can occupy 1 molecular orbital at a time. Furthermore, all orbitals at an energy level must be filled with one electron before they can be paired.  (see Figure 2 above)
4	Molecular orbitals are best formed when composed of Atomic orbitals of like energies.	Molecular Orbital Configuration of Li2: (?1s)2(?*1s)2(?2s)2 The bonding (?1s)2 and antibonding (?*1s)2 cancel each other out, leaving (?2s)2 as the valence electrons involved in the atoms' bonding. We use subscripts to indicate the atomic orbitals that have been combined. The asterisk (*) denotes an antibonding orbital.

Sigma Bonds Molecular orbitals that are symmetrical about the axis of the bond are called sigma molecular orbitals, often abbreviated by the Greek letter ?.  Figure 2 shows the 1s orbitals of 2 Hydrogen atoms forming a sigma orbital. There are two types of sigma orbitals formed, antibonding sigma orbitals (abbreviated ?*), and bonding sigma orbitals (abbreviated ?). In sigma bonding orbitals, the in phase atomic orbitals overlap end to end causing an increase in electron density along the bond axis. Where the atomic orbitals overlap, there is an increase in electron density and therefore an increase in the intensity of the negative charge. This increase in negative charge causes the nuclei to be drawn closer together. In sigma antibonding orbitals (?*), the out of phase 1s orbitals interfere destructively which results in a low electron density between the nuclei as seen on the top of the diagram. 

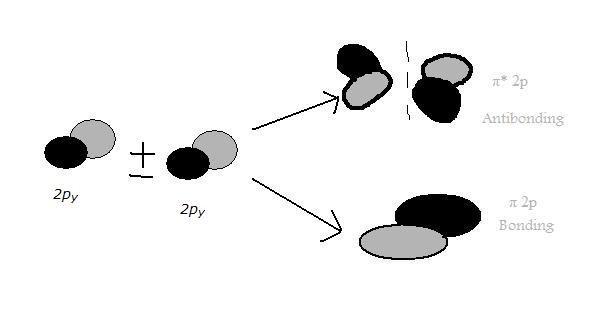
Figure 3 is a representation of the energy levels of the bonding and antibonding orbitals formed in the hydrogen molecule.  Two molecular orbitals were formed, one antibonding (?*) and one bonding (?).The two electrons in the hydrogen molecule have antiparallel spins. Notice that the ?* orbital is empty and has a higher energy than the ? orbital. 

Figure 3. Energy level diagram of H₂

For a description of Figure 3, click the figure.

Sigma bonding orbitals and antibonding orbitals can also be formed between p orbitals (shown below). Notice that as in previous examples, the p_x orbitals have to be in phase in order to form bonding molecular orbitals. Sigma molecular orbitals formed by p orbitals are often differentiated from other types of sigma orbitals by adding the subscript p below it. So the antibonding orbital shown in the diagram below would be ?*_p.

Figure 4. Molecular Orbitals from p Atomic Orbitals

For a description of Figure 4, click on the figure.
 

Pi Bonds

The pi bonding bonds as a side to side overlap (of the 2p_y or 2p_z), which then causes there to be no electron density along the axis (as in the overlap of 2p_x orbitals) , but there is density above and belong the axis. The diagram below shows a pi antibonding molecular orbital and a pi bonding molecular orbital.

Figure 5. Molecular Orbitals from p Atomic Orbitals Creating Pi Bonds

For a description of Figure 5, click on the figure

Figure 6. 2p_y Orbitals (see Figure 5 for youtube explination).

The two 2p_y atomic orbitals overlap parallel to form two pi molecular orbitals which are asymmetrical about the axis of the bond.

Figure 7. 2p_z orbitals(see Figure 5 for youtube explanation).

The two 2p_z orbitals overlap to create another pair of pi 2p and pi *2p molecular orbitals. The 2p_z-2p_z overlap is similar to the 2p_y-2p_y overlap because it is just the orbitals of the 2pz rotated 90 degrees about the axis. The new molecular orbitals have the same potential energies as those from the 2p_y-2p_y overlap.