Area of Triangle (three points)

The Area of a Triangle Based on Three Defined Points in a Plane calculator computes the area of a triangle given the coordinates (X_i, Y_i) of the triangle’s three vertices (P₁ , P₂ , P₃).

INSTRUCTIONS:  Enter the x and y coordinates of the triangle’s three vertices:

• (P₁) X and Y coordinates of vertex point 1
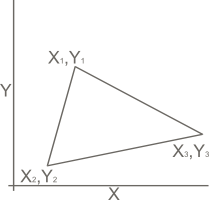
• (P₂) X and Y coordinates of vertex point 2
• (P₃) X and Y coordinates of vertex point 3

AREA (A): The calculator computes the area or the triangle.  Note: the units would be equal to those of the coordinates.

A triangle is a polygon with three sides, three vertices (corners), and three angles. Triangles can be classified based on the lengths of their sides and the measures of their angles as follows:

By Side Lengths:

• Equilateral Triangle: All three sides are equal in length.
• Isosceles Triangle: Two sides are equal in length.
• Scalene Triangle: All three sides have different lengths.

By Angle Measures:

• Acute Triangle: All three angles are less than 90 degrees.
• Right Triangle: One angle is exactly 90 degrees.
• Obtuse Triangle: One angle is greater than 90 degrees.

The sum of the interior angles of any triangle always adds up to 180 degrees. 

Triangle Calculators

• Area of Triangle (base and height)
• Area of Triangle (two sides and interior angle)
• Area of Triangle (two angles and interior side)
• Area of Triangle (three sides)
• Area of Equilateral Triangle
• Area of Triangle (three points)
• Height of Triangle
• Width of Triangle
• Triangle Perimeter
• Interior Angle of a triangle based on the length of three sides
• Semi-perimeter of a triangle
• Area of Circle Within a Triangle
• Area of Circle Around a Triangle
• Area between two vectors
• Triangle Volume