36.2 Angular momentum in three dimensions  by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.

36.2 Angular momentum in three dimensions

Up until now we've only worked with angular momentum in the context of rotation in a plane, for which we could simply use positive and negative signs to indicate clockwise and counterclockwise directions of rotation. A hydrogen atom, however, is unavoidably three-dimensional. Let's first consider the generalization of angular momentum to three dimensions in the classical case, and then consider how it carries over into quantum physics.

Three-dimensional angular momentum in classical physics

If we are to completely specify the angular momentum of a classical object like a top, b, in three dimensions, it's not 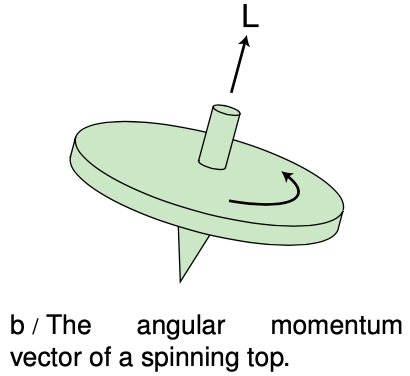enough to say whether the rotation is clockwise or counterclockwise. We must also give the orientation of the plane of rotation or, equivalently, the direction of the top's axis. The convention is to specify the direction of the axis. There are two possible directions along the axis, and as a matter of convention we use the direction such that if we sight along it, the rotation appears clockwise.

Angular momentum can, in fact, be defined as a vector pointing along this direction. This might seem like a strange definition, since nothing actually moves in that direction, but it wouldn't make sense to define the angular momentum vector as being in the direction of motion, because every part of the top has a different direction of motion. Ultimately it's not just a matter of picking a definition that is convenient and unambiguous: the definition we're using is the only one that makes the total angular momentum of a system a conserved quantity if we let “total” mean the vector sum.

As with rotation in one dimension, we cannot define what we mean by angular momentum in a particular situation unless we pick a point as an axis. This is really a different use of the word “axis” than the one in the previous paragraphs. Here we simply mean a point from which we measure the distance `r`. In the hydrogen atom, the nearly immobile proton provides a natural choice of axis.

Three-dimensional angular momentum in quantum physics

Once we start to think more carefully about the role of angular momentum in quantum physics, it may seem that there is a basic problem: the angular momentum of the electron in a hydrogen atom depends on both its distance from the proton and its momentum, so in order to know its angular momentum precisely it would seem we would need to know both its position and its momentum simultaneously with good accuracy. This, however, might seem to be forbidden by the Heisenberg uncertainty principle.

Actually the uncertainty principle does place limits on what can be known about a particle's angular momentum vector, but it does not prevent us from knowing its magnitude as an exact integer multiple of `?`. The reason is that in three dimensions, there are really three separate uncertainty principles:

`Deltap_xDeltax?h`

`Deltap_yDeltay?h`

`Deltap_zDeltaz?h`

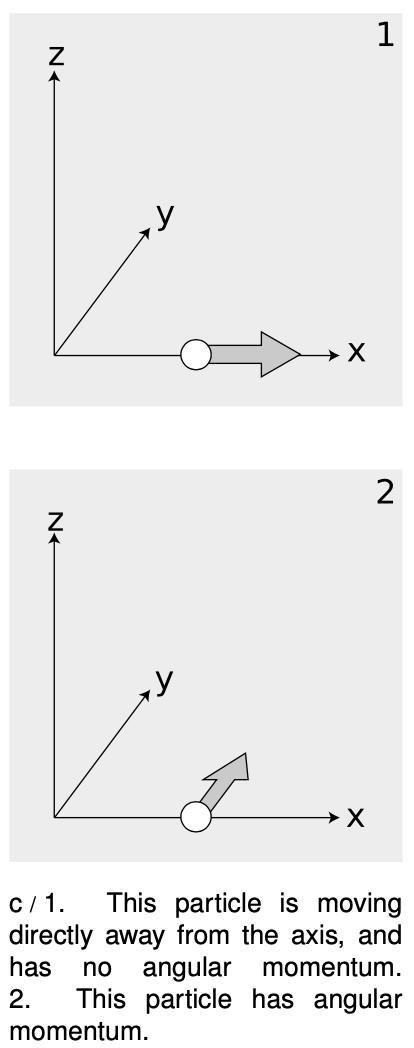
Now consider a particle, c/1, that is moving along the xx axis at position xx and with momentum pxpx. We may not be able to know both `x` and `p_x` with unlimited accuracy, but we can still know the particle's angular momentum about the origin exactly. Classically, it is zero, because the particle is moving directly away from the origin: if it was to be nonzero, we would need both a nonzero `x` and a nonzero `p_y`. In quantum terms, the uncertainty principle does not place any constraint on `DeltaxDeltap_y`.

Suppose, on the other hand, a particle finds itself, as in figure c/2, at a position `x` along the `x` axis, and it is moving parallel to the `y` axis with momentum pypy. It has angular momentum `xp_y` about the `z` axis, and again we can know its angular momentum with unlimited accuracy, because the uncertainty principle only relates `x` to `p_x` and `y` to `p_y`. It does not relate `x` to `p_y`.

As shown by these examples, the uncertainty principle does not restrict the accuracy of our knowledge of angular momenta as severely as might be imagined. However, it does prevent us from knowing all three components of an angular momentum vector simultaneously. The most general statement about this is the following theorem, which we present without proof:

the angular momentum vector in quantum physics

36.2 Angular momentum in three dimensions  by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.