21.2 Electrical forces by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license. 

21.2 Electrical forces

Charge

“Charge” is the technical term used to indicate that an object has been prepared so as to participate in electrical forces. This is to be distinguished from the common usage, in which the term is used indiscriminately for anything electrical. For example, although we speak colloquially of “charging” a battery, you may easily verify that a battery has no charge in the technical sense, e.g., it does not exert any electrical force on a piece of tape that has been prepared as described in the previous section.

Two types of charge

We can easily collect reams of data on electrical forces between different substances that have been charged in different ways. We find for example that cat fur prepared by rubbing against rabbit fur will attract glass that has been rubbed on silk. How can we make any sense of all this information? A vast simplification is achieved by noting that there are really only two types of charge. Suppose we pick cat fur rubbed on rabbit fur as a representative of type A, and glass rubbed on silk for type B. We will now find that there is no “type C.” Any object electrified by any method is either A-like, attracting things A attracts and repelling those it repels, or B-like, displaying the same attractions and repulsions as B. The two types, A and B, always display opposite interactions. If A displays an attraction with some charged object, then B is guaranteed to undergo repulsion with it, and vice-versa.

The coulomb

Although there are only two types of charge, each type can come in different amounts. The metric unit of charge is the coulomb (rhymes with “drool on”), defined as follows:

One Coulomb (C) is the amount of charge such that a force of `9.0×10^9` N occurs between two point-like objects with charges of 1 C separated by a distance of 1 m.

The notation for an amount of charge is `q`. The numerical factor in the definition is historical in origin, and is not worth memorizing. The definition is stated for point-like, i.e., very small, objects, because otherwise different parts of them would be at different distances from each other.

A model of two types of charged particles

Experiments show that all the methods of rubbing or otherwise charging objects involve two objects, and both of them end up getting charged. If one object acquires a certain amount of one type of charge, then the other ends up with an equal amount of the other type. Various interpretations of this are possible, but the simplest is that the basic building blocks of matter come in two flavors, one with each type of charge. Rubbing objects together results in the transfer of some of these particles from one object to the other. In this model, an object that has not been electrically prepared may actually possesses a great deal of both types of charge, but the amounts are equal and they are distributed in the same way throughout it. Since type A repels anything that type B attracts, and vice versa, the object will make a total force of zero on any other object. The rest of this chapter fleshes out this model and discusses how these mysterious particles can be understood as being internal parts of atoms.

Use of positive and negative signs for charge

Because the two types of charge tend to cancel out each other's forces, it makes sense to label them using positive and negative signs, and to discuss the total charge of an object. It is entirely arbitrary which type of charge to call negative and which to call positive. Benjamin Franklin decided to describe the one we've been calling “A” as negative, but it really doesn't matter as long as everyone is consistent with everyone else. An object with a total charge of zero (equal amounts of both types) is referred to as electrically neutral.

self-check:

Criticize the following statement: “There are two types of charge, attractive and repulsive.”

(answer in the back of the PDF version of the book)

Coulomb's law

A large body of experimental observations can be summarized as follows:

Clever modern techniques have allowed the `1"/"r^2` form of Coulomb's law to be tested to incredible accuracy, showing that the exponent is in the range from `1.9999999999999998` to `2.0000000000000002`.

Note that Coulomb's law is closely analogous to Newton's law of gravity, where the magnitude of the force is `Gm_1m_2"/"r^2`, except that there is only one type of mass, not two, and gravitational forces are never repulsive. Because of this close analogy between the two types of forces, we can recycle a great deal of our knowledge of gravitational forces. For instance, there is an electrical equivalent of the shell theorem: the electrical forces exerted externally by a uniformly charged spherical shell are the same as if all the charge was concentrated at its center, and the forces exerted internally are zero.

Conservation of charge

An even more fundamental reason for using positive and negative signs for electrical charge is that experiments show that charge is conserved according to this definition: in any closed system, the total amount of charge is a constant. This is why we observe that rubbing initially uncharged substances together always has the result that one gains a certain amount of one type of charge, while the other acquires an equal amount of the other type. Conservation of charge seems natural in our model in which matter is made of positive and negative particles. If the charge on each particle is a fixed property of that type of particle, and if the particles themselves can be neither created nor destroyed, then conservation of charge is inevitable.

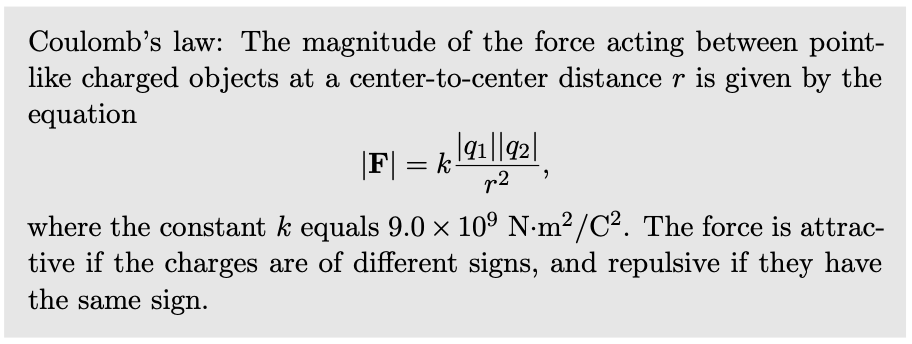
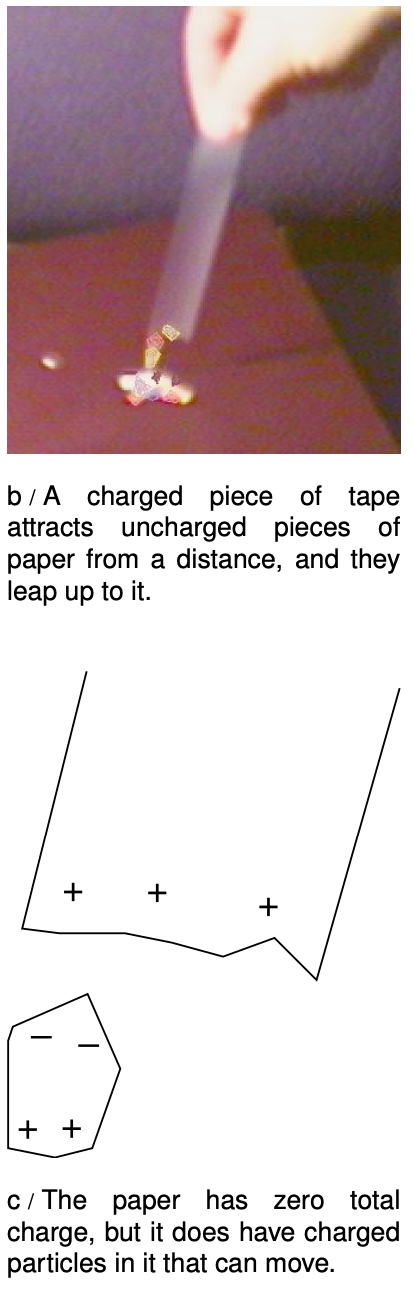Electrical forces involving neutral objects

As shown in figure b, an electrically charged object can attract objects that are uncharged. How is this possible? The key is that even though each piece of paper has a total charge of zero, it has at least some charged particles in it that have some freedom to move. Suppose that the tape is positively charged, c. Mobile particles in the paper will respond to the tape's forces, causing one end of the paper to become negatively charged and the other to become positive. The attraction between the paper and the tape is now stronger than the repulsion, because the negatively charged end is closer to the tape.

self-check:

What would have happened if the tape was negatively charged?

(answer in the back of the PDF version of the book)

Discussion Questions

• A - If the electrical attraction between two point-like objects at a distance of 1 m is `9×10^9` N, why can't we infer that their charges are `+1` and `-1` C? What further observations would we need to do in order to prove this?
• B - An electrically charged piece of tape will be attracted to your hand. Does that allow us to tell whether the mobile charged particles in your hand are positive or negative, or both?

21.2 Electrical forces by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.