5.5 Objects under strain by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.

5.5 Objects under strain

A string lengthens slightly when you stretch it. Similarly, we have already discussed how an apparently rigid object such as a wall is actually flexing when it participates in a normal force. In other cases, the effect is more obvious. A spring or a rubber band visibly elongates when stretched.

Common to all these examples is a change in shape of some kind: lengthening, bending, compressing, etc. The change in shape can be measured by picking some part of the object and measuring its position, `x`. For concreteness, let's imagine a spring with one end attached to a wall. When no force is exerted, the unfixed end of the spring is at some position `x_o`. If a force acts at the unfixed end, its position will change to some new value of `x`. The more force, the greater the departure of `x` from `x_o`.

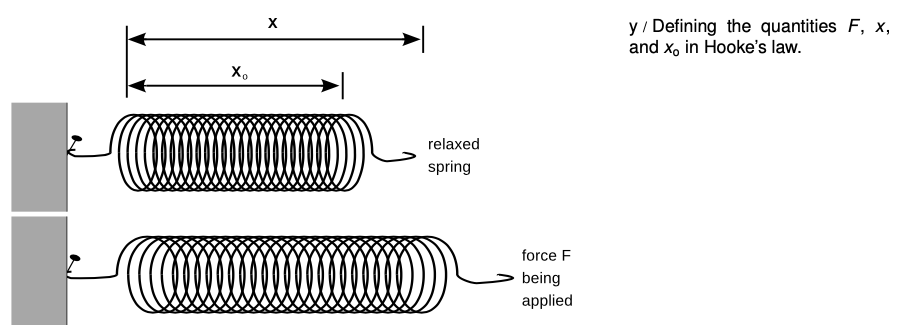
Back in Newton's time, experiments like this were considered cutting-edge research, and his contemporary Hooke is remembered today for doing them and for coming up with a simple mathematical generalization called Hooke's law:

`F \approx k(x-x_o)`  [force required to stretch a spring; valid for small forces only]

Here `k` is a constant, called the spring constant, that depends on how stiff the object is. If too much force is applied, the spring exhibits more complicated behavior, so the equation is only a good approximation if the force is sufficiently small. Usually when the force is so large that Hooke's law is a bad approximation, the force ends up permanently bending or breaking the spring.

Although Hooke's law may seem like a piece of trivia about springs, it is actually far more important than that, because all solid objects exert Hooke's-law behavior over some range of sufficiently small forces. For example, if you push down on the hood of a car, it dips by an amount that is directly proportional to the force. (But the car's behavior would not be as mathematically simple if you dropped a boulder on the hood!)

`=>` Solved problem: Combining springs — problem 14

`=>` Solved problem: Young's modulus — problem 16

Discussion Question

`bb"A"`     A car is connected to its axles through big, stiff springs called shock absorbers, or “shocks.” Although we've discussed Hooke's law above only in the case of stretching a spring, a car's shocks are continually going through both stretching and compression. In this situation, how would you interpret the positive and negative signs in Hooke's law?

5.5 Objects under strain by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.