Hemicylinder Optics Lab

Last modified by

on

Jul 24, 2020, 6:28:07 PM

Created by

on

May 24, 2016, 4:29:25 AM

θ_{t} = \sin^{- 1} (\frac{1}{n_{2}} \sin (θ_{i}))

$theta_t = sin^(-1)((1)/(n_2) sin( theta_i ))$

θ_{i}

$theta_i$

Return data in...

$"Return data in..."$

Pre-Lab

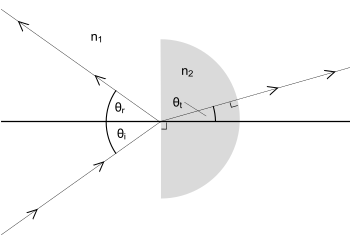/attachments/188dbd45-2168-11e6-9770-bc764e2038f2/HemicylinderOptics.png Top-down view of the lab setup For this experiment, you would need a laser and a plastic hemicylinder. The laser emits a beam of light that takes the path shown in the picture. The laser strikes the hemicylinder in the middle of its flat side. The picture is a top-down view, so it looks like the hemicylinder is a semi-circle. The hemicylinder is a three-dimensional shape, so it does have a thickness. However, we can ignore that thickness as long as the laser and hemicylinder are level (which they are, for this experiment).

Where did we get this equation

Snell's Law (the Law of Refraction) is $n_{1} \sin (θ_{i}) = n_{2} \sin (θ_{t})$ $n_1 sin(theta_i) = n_2 sin(theta_t)$ . If we rearrange this equation to solve for $θ_{t}$ $theta_t$ , we get that $θ_{t} = \sin^{- 1} (\frac{n_{1}}{n_{2}} \sin (θ_{i}))$ $theta_t = sin^(-1) (n_1/n_2 sin(theta_i))$ . This experiment assumes that the beam of light is going from air into the plastic hemicylinder. The index of refraction of air ( $n_{a i r}$ $n_(air)$ ) is very close to 1, so we can just substitute 1 in for $n_{1}$ $n_1$ and we achieve the above equation: $θ_{t} = \sin^{- 1} (\frac{1}{n_{2}} \sin (θ_{i}))$ $theta_t = sin^(-1) (1/n_2 sin(theta_i))$

How to use the equation

Simply enter the incident angle and select whether you'd like your answer in degrees or radians (degrees is the default). The equation only shows the formula for $θ_{t}$ $theta_t$ because it's the most complex. For more information regarding the law of reflection, you can look at this page.

You might notice that if you enter, say, 15 degrees twice, you'll get two different answers. This equation has some "experimental noise" built into it. In a real lab, your results will be different from what's theoretically expected. That's not because the theory is necessarily wrong, but rather because we can't run a perfect experiment. To try and cut through the experimental noise, scientists generally use large data sets and statistics. In a more casual lab, such as this one, the "statistical analysis" is just an average of a handful of data points. Is it perfectly rigorous? No. But hopefully it gets you used to how scientific experiments work.

Lab Exercise

For this lab, I recommend using a table like the following:

$θ_{i}$ $theta_i$	$θ_{r}$ $theta_r$	$θ_{t}$ $theta_t$	$n_{2}$ $n_2$
0°
15°
30°
45°
60°
75°
90°

This is just a suggestion. You may use more or different incident angles if you wish (I would caution against using fewer incident angles since fewer data points generally means an experiment is less accurate). You may also measure the angles in radians, if you wish.

Estimating n₂

The index of refraction of air is (very close to) 1, but what about the index of refraction of this plastic hemicylinder? The equation at the top of this page doesn't show you what the value is for $n_{2}$ $n_2$ , but it is built into the code for this page. You're going to try and estimate the value of $n_{2}$ $n_2$ by using the relationship between the incident angle and transmitted angle. We know that $n_{1} \sin (θ_{i}) = n_{2} \sin (θ_{t})$ $n_1 sin(theta_i) = n_2 sin(theta_t)$ . We also know $n_{1}$ $n_1$ , and we have many pairs of incident and transmitted angles. This will allow us to solve for many approximate values of $n_{2}$ $n_2$ . If the math is giving you trouble, try this calculator/ (Note: $θ_{i} = 0$ $theta_i = 0$ won't help us find $n_{2}$ $n_2$ since normal incidence results in $θ_{t} = 0$ $theta_t = 0$ no matter what $n_{2}$ $n_2$ is.)

Once you have your approximate values of $n_{2}$ $n_2$ , average them. Below your table (or whatever you're using to keep track of the data), write "Average experimental value of $n_{2}$ $n_2$ : " and then the average.

Why a Hemicylinder?

In a laboratory setting, we can't easily examine the light while it's still inside the hemicylinder. Instead, we must wait for the light to leave the hemicylinder and strike a surface before we can tell where it is. With that in mind, why did we choose a hemicylinder instead of a cube, sphere, or random blob? Give your best guess. Hint: it's very important that the laser strikes the middle of the hemicylinder's flat side.

Error Analysis

This lab simulation's error is artificially built in, but live experiments have reasons for error. Imagine that you're actually performing this experiment. You have your laser and hemicylinder set up. You send the light into the hemicylinder, and then measure the angles of reflection and refraction. You repeat this for a variety of incident angles. What are two potential sources of error that would result in your answers being different from what's theoretically expected?

This lab simulation is based on an actual lab exercise from Professor Charles Adler at St. Mary's College of Maryland.

This equation, Hemicylinder Optics Lab, is used in 1 page

Calculators

Equations and Data Items

Collections

• Physics Collection by TylerJones

Comments
Attachments
Stats

No comments

HemicylinderOptics.png

Last 12 Months

This site uses cookies to give you the best, most relevant experience. By continuing to browse the site you are agreeing to our use of cookies.

Hemicylinder Optics Lab

Pre-Lab

Where did we get this equation

How to use the equation

Lab Exercise

Why a Hemicylinder?

Error Analysis

Datasets

Equations and Data Items

Calculators

Equations and Data Items

Collections