The Object Weight calculator contains the mass / weight equations for a variety of geometric shapes and provides the mean density for a large number of substances.   By combining these you can estimate the mass or weight of an object.  More simply stated, if you know the shape of an object, its size (dimensions) and what it's made of, you should be able to make a good estimate of its mass or weight.  This calculator helps you do precisely that.

The Mass of Shapes equations in this calculator let the user:

1. Choose a geometric shape or container type (see diagrams below),
2. enter the dimensions of the object, and
3. enter a mean density to calculate the object's approximate mass.  Assuming nominal Earth gravity, the mass can be converted to nominal Earth surface weight (e.g. pounds from kilograms). 
4. If you don't know the mean density of the content of the shape, first go to the `mu` Density Constants tab.  There are several common substances listed.  If you do not find yours, click on the mean Density Lookup button and scroll through the 500+ choices to find the mean density of your substance or something close.  Use that mean density number in the geometric shape equations to compute the mass/weight.

vCalc provides a lookup function that contains the mean density in kilograms per cubic meter of hundreds of substances from farm commodities (e.g. grains, woods, etc) to quary items (types of stone and sand) to commonly transported liquids (e.g. water, diesel). 

The basic formula for all of the equations is this:

                           ` "mass" = mu"Density" * "volume"`

Geometric Shapes or Containers

• Cube, Box, Cone, Cone (frustum), Cylinder, Slanted Cylinder,
• Ellipsoid, Capsule, Bottle, Paraboloid, Pyramid, Pyramid Frustum, 
• Sphere, Sphere Cap, Sphere Segment, Prism, Torus, Chamfer 

Diagrams of these objects are below.

Mean Density Table

Mean density is scientifically volume divided by mass.  There are  various unit for density adopted by cultures and industries. Common units for density included the following:

• kilograms per cubic meter (kg/m³)
• grams per cubic centimeter (g/cm³)
• grams per liter (g/L)
• pounds per cubic feet (lb/ft³)
• ounces per cubic inch (oz/in³)
• pounds per barrel (lb/bbl)
• pounds per bushel (lb/bu)

If you want to identify a material by its density, use the Density Within Range tool.

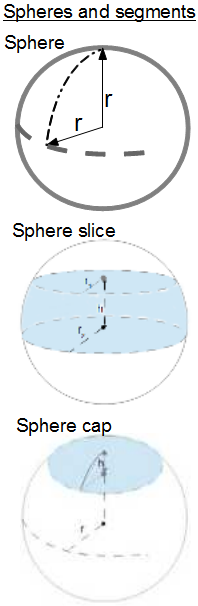spheres 

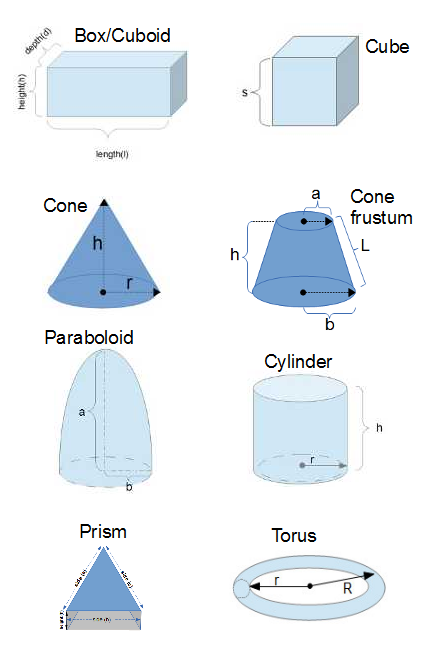   various shapes various frustums

See Also

• Moments of Inertia  - calculator for the MoI of different shapes around different axes.
• Geometry (3D) - calculator for euclidean objects
• Volume - Volume calculator for various geometric shapes.
• Trucking calculator - Load calculator