7.6 Summary by Benjamin Crowell, Light and Matter licensed under the Creative Commons Attribution-ShareAlike license.
Summary
Vocabulary
vector — a quantity that has both an amount (magnitude) and a direction in space
magnitude — the “amount” associated with a vector
scalar — a quantity that has no direction in space, only an amount
Notation
`A`................................... a vector with components `A_x`, `A_y`, and `A_z`
`vecA`.................................. a handwritten notation for a vector
`|A|`................................. the magnitude of vector `A`
`r`.................................... the vector whose components are `x`, `y`, and `z`
`Deltar`................................ the vector whose components are `Deltax`, `Deltay`, and `Deltaz`
`hatx`, `haty`, `hatz`.......................... (optional topic) unit vectors; the vectors with magnitude 1 lying along the `x`, `y`, and `z` axes
`hati`,`hath`,`hatk`............................. a harder to remember notation for the unit vectors
Other Terminology and Notation
displacement vector...... a name for the symbol `Deltar`
speed............................ the magnitude of the velocity vector, i.e., the velocity stripped of any information about its direction
Summary
A vector is a quantity that has both a magnitude (amount) and a direction in space, as opposed to a scalar, which has no direction. The vector notation amounts simply to an abbreviation for writing the vector's three components.
In two dimensions, a vector can be represented either by its two components or by its magnitude and direction. The two ways of describing a vector can be related by trigonometry.
The two main operations on vectors are addition of a vector to a vector, and multiplication of a vector by a scalar.
Vector addition means adding the components of two vectors to form the components of a new vector. In graphical terms, this corresponds to drawing the vectors as two arrows laid tip-to-tail and drawing the sum vector from the tail of the first vector to the tip of the second one. Vector subtraction is performed by negating the vector to be subtracted and then adding.
Multiplying a vector by a scalar means multiplying each of its components by the scalar to create a new vector. Division by a scalar is defined similarly.
Homework Problems
Key
`sqrt` A computerized answer check is available online.
`int` A problem that requires calculus.
`***` A difficult problem.
1. The figure shows vectors `A` and `B`. As in figure h on p. 208, graphically calculate the following:
`A+B`, `A-B`, `B-A`, `-2B`, `A-2B`
No numbers are involved.
2. Phnom Penh is 470 km east and 250 km south of Bangkok. Hanoi is 60 km east and 1030 km north of Phnom Penh.
(a) Choose a coordinate system, and translate these data into `Deltax` and `Deltay` values with the proper plus and minus signs.
(b) Find the components of the `Deltar` vector pointing from Bangkok to Hanoi. `sqrt`
3. If you walk 35 km at an angle `25°` counterclockwise from east, and then 22 km at `230°` counterclockwise from east, find the distance and direction from your starting point to your destination. `sqrt`
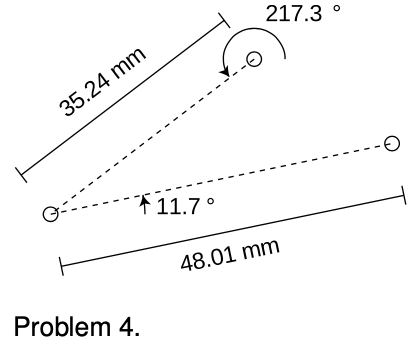4. A machinist is drilling holes in a piece of aluminum according to the plan shown in the figure. She starts with the top hole, then moves to the one on the left, and then to the one on the right. Since this is a high-precision job, she finishes by moving in the direction and at the angle that should take her back to the top hole, and checks that she ends up in the same place. What are the distance and direction from the right-hand hole to the top one? `sqrt`
5. Suppose someone proposes a new operation in which a vector `A` and a scalar `B` are added together to make a new vector `C` like this:
`C_x=A_x+B`
`C_y=A_y+B`
`C_z=A_z+B`
Prove that this operation won't be useful in physics, because it's not rotationally invariant.
7.6 Summary by Benjamin Crowell, Light and Matter licensed under the Creative Commons Attribution-ShareAlike license.