32.7 Single-slit diffraction by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.

32.7 Single-slit diffraction

If we use only a single slit, is there diffraction? If the slit is not wide compared to a wavelength of light, then we can approximate its behavior by using only a single set of Huygens ripples. There are no other sets of ripples to add to it, so there are no constructive or destructive interference effects, and no maxima or minima. The result will be a uniform spherical wave of light spreading out in all directions, like what we would expect from a tiny lightbulb. We could call this a diffraction pattern, but it is a completely featureless one, and it could not be used, for instance, to determine the wavelength of the light, as other diffraction patterns could.

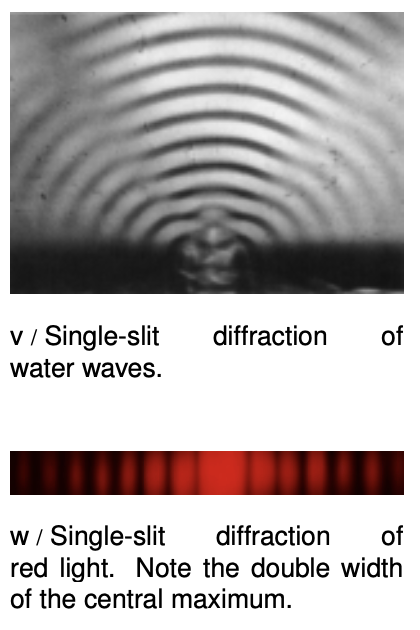
All of this, however, assumes that the slit is narrow compared to a wavelength of light. If, on the other hand, the slit is broader, there will indeed be interference among the sets of ripples spreading out from various points along the opening. Figure v shows an example with water waves, and figure w with light.

self-check:

How does the wavelength of the waves compare with the width of the slit in figure v?

(answer in the back of the PDF version of the book)

We will not go into the details of the analysis of single-slit diffraction, but let us see how its properties can be related to the general things we've learned about diffraction. We know based on scaling arguments that the angular sizes of features in the diffraction pattern must be related to the wavelength and the width, `a`, of the slit by some relationship of the form

`lambda/aharrtheta`.

This is indeed true, and for instance the angle between the maximum of the central fringe and the maximum of the next fringe on one side equals `1.5lambda"/"a`. Scaling arguments will never produce factors such as the `1.5`, but they tell us that the answer must involve `lambda"/"a`, so all the familiar qualitative facts are true. For instance, shorter-wavelength light will produce a more closely spaced diffraction pattern.

An important scientific example of single-slit diffraction is in telescopes. Images of individual stars, as in figure y, are a good way to examine diffraction effects, because all stars except the sun are so far away that no telescope, even at the highest magnification, can image their disks or surface features. Thus any features of a star's image must be due purely to optical effects such as diffraction. A prominent cross appears around the brightest star, and dimmer ones surround the dimmer stars. Something like this is seen in most telescope photos, and indicates that inside the tube of the telescope there were two perpendicular struts or supports. Light diffracted around these struts. You might think that diffraction could be eliminated entirely by getting rid of all obstructions in the tube, but the circles around the stars are diffraction effects arising from single-slit diffraction at the mouth of the telescope's tube! (Actually we have not even talked about diffraction through a circular opening, but the idea is the same.) Since the angular sizes of the diffracted images depend on `lambda"/"a`, the only way to improve the resolution of the images is to increase the diameter, `a`, of the tube. This is one of the main reasons (in addition to light-gathering power) why the best telescopes must be very large in diameter.

self-check:

What would this imply about radio telescopes as compared with visible-light telescopes?

(answer in the back of the PDF version of the book)

Double-slit diffraction is easier to understand conceptually than single-slit diffraction, but if you do a double-slit diffraction experiment in real life, you are likely to encounter a complicated pattern like figure aa/1, rather than the simpler one, 2, you were expecting. This is because the slits are fairly big compared to the wavelength of the light being used. We really have two different distances in our pair of slits: `d`, the distance between the slits, and `w`, the width of each slit. Remember that smaller distances on the object the light diffracts around correspond to larger features of the diffraction pattern. The pattern 1 thus has two spacings in it: a short spacing corresponding to the large distance `d`, and a long spacing that relates to the small dimension `w`.

Discussion Question

A   Why is it optically impossible for bacteria to evolve eyes that use visible light to form images?

32.7 Single-slit diffraction by Benjamin Crowell, Light and Matter  licensed under the Creative Commons Attribution-ShareAlike license.