1.4 Order-of-magnitude estimates by Benjamin Crowell, Light and Matter licensed under the Creative Commons Attribution-ShareAlike license.
1.4 Order-of-magnitude estimates
It is the mark of an instructed mind to rest satisfied with the degree of precision that the nature of the subject permits and not to seek an exactness where only an approximation of the truth is possible. --Aristotle
It is a common misconception that science must be exact. For instance, in the Star Trek TV series, it would often happen that Captain Kirk would ask Mr. Spock, “Spock, we're in a pretty bad situation. What do you think are our chances of getting out of here?” The scientific Mr. Spock would answer with something like, “Captain, I estimate the odds as 237.345 to one.” In reality, he could not have estimated the odds with six significant figures of accuracy, but nevertheless one of the hallmarks of a person with a good education in science is the ability to make estimates that are likely to be at least somewhere in the right ballpark. In many such situations, it is often only necessary to get an answer that is off by no more than a factor of ten in either direction. Since things that differ by a factor of ten are said to differ by one order of magnitude, such an estimate is called an order-of-magnitude estimate. The tilde, ~ , is used to indicate that things are only of the same order of magnitude, but not exactly equal, as in
odds of survival ~ 100 to one.
The tilde can also be used in front of an individual number to emphasize that the number is only of the right order of magnitude.
Although making order-of-magnitude estimates seems simple and natural to experienced scientists, it's a mode of reasoning that is completely unfamiliar to most college students. Some of the typical mental steps can be illustrated in the following example.
Example 4: Cost of transporting tomatoes (incorrect solution)
`=>` Roughly what percentage of the price of a tomato comes from the cost of transporting it in a truck?
`=>` The following incorrect solution illustrates one of the main ways you can go wrong in order-of-magnitude estimates.
Incorrect solution: Let's say the trucker needs to make a $400 profit on the trip. Taking into account her benefits, the cost of gas, and maintenance and payments on the truck, let's say the total cost is more like $2000. I'd guess about 5000 tomatoes would fit in the back of the truck, so the extra cost per tomato is 40 cents. That means the cost of transporting one tomato is comparable to the cost of the tomato itself. Transportation really adds a lot to the cost of produce, I guess.
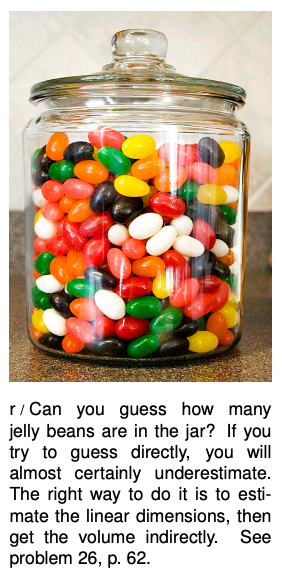The problem is that the human brain is not very good at estimating area or volume, so it turns out the estimate of 5000 tomatoes fitting in the truck is way off. That's why people have a hard time at those contests where you are supposed to estimate the number of jellybeans in a big jar. Another example is that most people think their families use about 10 gallons of water per day, but in reality the average is about 300 gallons per day. When estimating area or volume, you are much better off estimating linear dimensions, and computing volume from the linear dimensions. Here's a better solution to the problem about the tomato truck:
Example 5: Cost of transporting tomatoes (correct solution)
As in the previous solution, say the cost of the trip is $2000. The dimensions of the bin are probably 4 m × 2 m × 1 m, for a volume of 8 m3. Since the whole thing is just an order-of-magnitude estimate, let's round that off to the nearest power of ten, 10 m3. The shape of a tomato is complicated, and I don't know any formula for the volume of a tomato shape, but since this is just an estimate, let's pretend that a tomato is a cube, 0.05 m × 0.05 m × 0.05 m, for a volume of 1.25×10-4 m3. Since this is just a rough estimate, let's round that to 10-4 m3. We can find the total number of tomatoes by dividing the volume of the bin by the volume of one tomato: `10 "m"^3"/"10-4 "m"^3=105` tomatoes. The transportation cost per tomato is `$2000"/"10^5` tomatoes = `$0.02`/tomato. That means that transportation really doesn't contribute very much to the cost of a tomato.
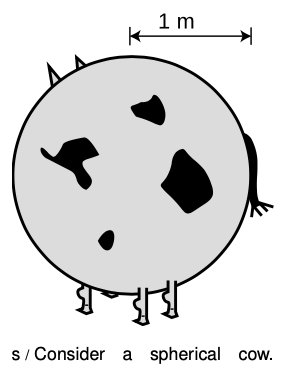Approximating the shape of a tomato as a cube is an example of another general strategy for making order-of-magnitude estimates. A similar situation would occur if you were trying to estimate how many `"m"^2` of leather could be produced from a herd of ten thousand cattle. There is no point in trying to take into account the shape of the cows' bodies. A reasonable plan of attack might be to consider a spherical cow. Probably a cow has roughly the same surface area as a sphere (`SA = 4 pi r^2`) with a radius of about `1 "m"`, which would be `4pi(1 "m")^2`. Using the well-known facts that pi equals three, and four times three equals about ten, we can guess that a cow has a surface area of about `10 "m"^2`, so the herd as a whole might yield `105 "m"^2`of leather.
Example 6: Estimating mass indirectly
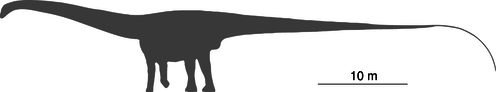
Usually the best way to estimate mass is to estimate linear dimensions, then use those to infer volume, and then get the mass based on the volume. For example, Amphicoelias, shown in the figure, may have been the largest land animal ever to live. Fossils tell us the linear dimensions of an animal, but we can only indirectly guess its mass. Given the length scale in the figure, let's estimate the mass of an Amphicoelias.
Its torso looks like it can be approximated by a rectangular box with dimensions `10 "m"×5 "m"×3 "m"` (`Vol = l * w * d`), giving about `2×102 "m"^3`. Living things are mostly made of water, so we assume the animal to have the density of water, `1 "g/cm"^3`, which converts to `103 "kg/m"^3`. This gives a mass of about `2×10^5 "kg"`, or `200` metric tons.
The following list summarizes the strategies for getting a good order-of-magnitude estimate.
1. Don't even attempt more than one significant figure of precision.
2. Don't guess area, volume, or mass directly. Guess linear dimensions and get area, volume, or mass from them.
3. When dealing with areas or volumes of objects with complex shapes, idealize them as if they were some simpler shape, a cube or a sphere, for example.
4. Check your final answer to see if it is reasonable. If you estimate that a herd of ten thousand cattle would yield `0.01 "m"^2` of leather, then you have probably made a mistake with conversion factors somewhere.
1.4 Order-of-magnitude estimates by Benjamin Crowell, Light and Matter licensed under the Creative Commons Attribution-ShareAlike license.