vector angle (2D)

The vector angle (2D) formula, Θ = tan^-1(Δy/Δx), computes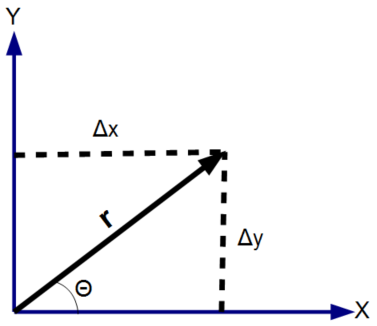  Vector r  components the angle between the vector r and the x axis based on the measurement of the two components of the two dimensional vector.

INSTRUCTIONS: Enter the following:

• (Δy) y coordinate.
• (Δx) x coordinate.

vector angle (Θ): The calculator returns the angle in degree.  However, this can be automatically converted to other angle units via the pull-down menu.

References

• Light and Matter by Benjamin Crowell, Chapter 7.2 Calculations ith magnitude and direction

Vectors

A vector is a mathematical concept of an object that has both direction and length.  A line alone is not a vector but a line with directed orientation spanning the distance between two points in space is a vector.  This equation computes the component of a vector that is the vector's projection on the x-axis of Cartesian coordinates.

The Math / Science

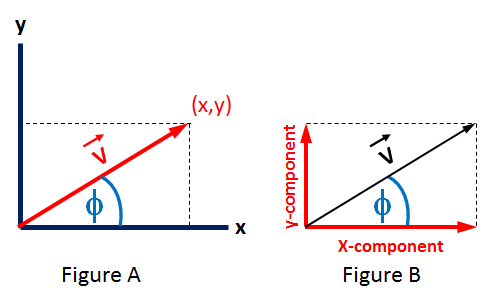
Figure A shows a vector, `bar V` (in red) represented in Cartesian coordinates.  A vector can point in any direction, so there is no significance to the fact that `bar V` in Figure A is pointing to a point in the +x/+y quadrant of the Cartesian coordinates. 

The direction and length of `bar V` define it as a vector and the vector can be displaced to any point in space and still be vector `bar V`. The beginning of the vector does not have to be located at the intersection of the x and y coordinates. We show vector `bar V`, with it's beginning point at the origin to simplify the idea that the x-component is the projection of the vector onto the x-axis. The angle, `phi`, between the x-axis and the vector, `bar V`, is used in this equation to compute the x-component of the vector, the projection of the vector, `bar V` onto the x-axis.

Figure B shows the Cartesian x and y components of the vector `bar V`.  Figure B shows the vector `bar V` displaced from the vector shown in Figure A but the two vectors could be considered equivalent because they have the same length and they both point in the same direction.

See also

V2 - Vector (y-component)