Tags | |
UUID | 5ae83e98-b451-11ec-be52-bc764e203090 |
A SIMPLE CAPACITANCE CALCULATOR
This calculator can be used to calculate the capacitance of a capacitor, series capacitance, and parallel capacitance.
What is capacitance?
Capacitance is a measure of the capacitor’s ability to store charge.
Capacitors are devices used to store large amounts of electrical energy. A capacitor consists of two conducting surfaces (usually metal plates) separated by an insulating material such as air, or paper. The insulating material is called dielectric.
SI unit of capacitance
The SI unit of capacitance is Farad.
The sub-units of Farad are:
- Kilofarad
- Milifarad
- Microfarad
- Nanofarad
- Picofarad
| Name | Abbreviations | Weight | Farads |
|---|---|---|---|
| Kilofarad | kF | 103 | 1000F |
| Milifarad | mF | 10-3 | 0.001F |
| Microfarad | µF | 10-6 | 0.000001F |
| Nanofarad | nF | 10-9 | 0.000000001F |
| Picofarad | pF | 10-12 | 0.000000000001F |
Example:
Convert the following values:
1. 0.5µF to nF
1×103 µF = 1nF
0.5 x 103= 500nF
2. 600pF to µF
1 ×10-6pF = 1µF
600 × (1×10-6) = 0.0006µF
3. 22nF to F
1×10-9 nF = 1F
22 × (1×10-9) = 0.000000022F
Capacitance Formula
The basic equation for capacitance is:
C=Q/V
Where
C=Capacitance in Farads
Q=Charge held on plates in coulombs
V=Potential difference across plates in Volts
To be able to remember the formula easily we can use the capacitance memory triangle.

(Image source: electronics-tutorials)
From the memory triangle above we can come up with three equations:
(Image source: Electronicnotes)
Capacitor Symbol
In a diagram, capacitance is expressed by the image below:
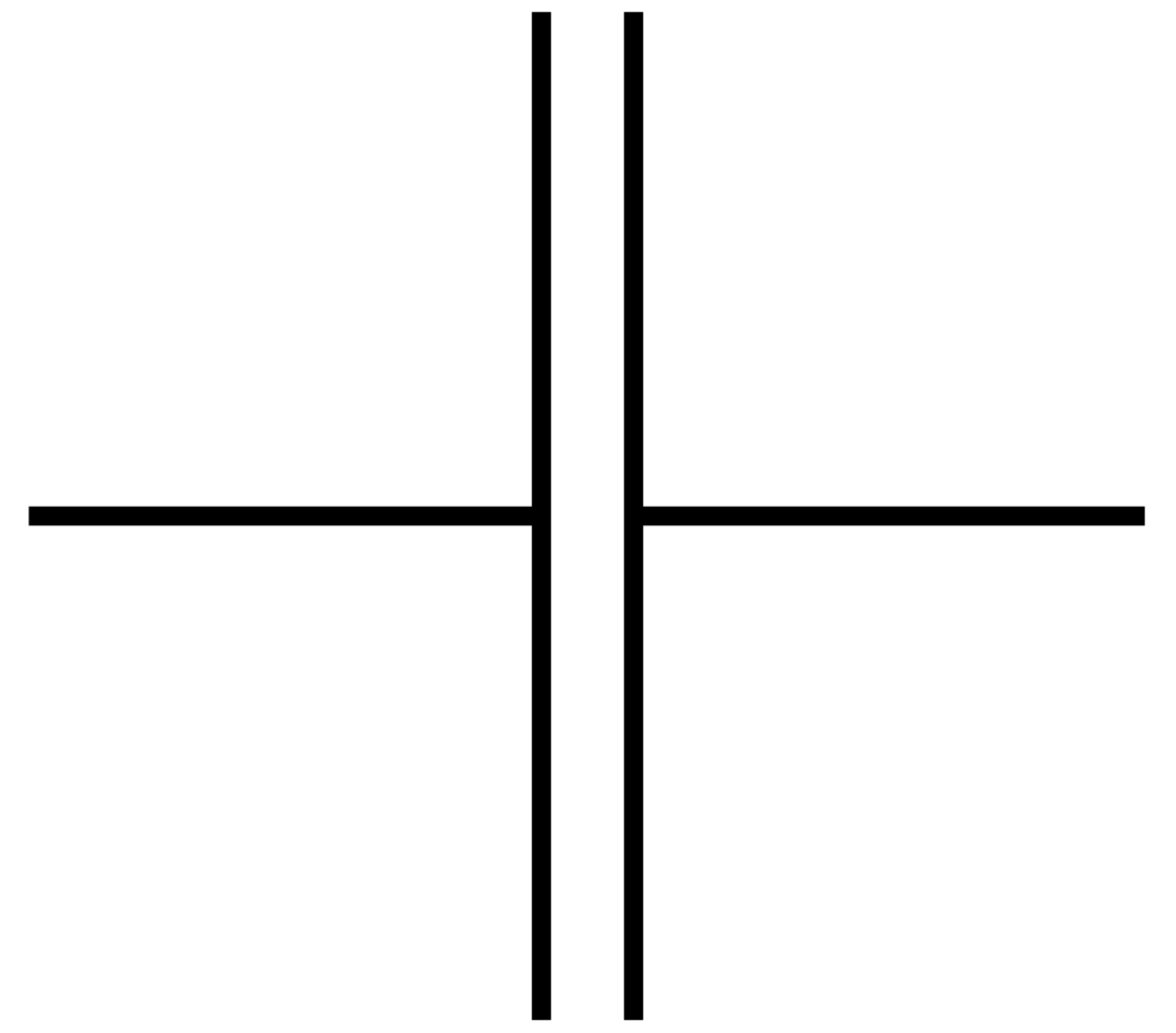
Parallel Capacitance

(Image source:Lambdageeks.com)
To calculate capacitors in parallel use the equation:
Cn=C1+C2+C3
EXAMPLE
Calculate the total capacitance in the circuit above where C1=12F, C2=20F, and C3=2F
Cn=C1+C2+C3
C=12F+20F+2F
=34F
Series Capacitance

(Image source:electrical4u.com)
The formula is:
1/Cn=1/C1+1/C2+1/C3
Calculate the total capacitance in the circuit above where C1=21F, C2=10F, and C3=12F
1/Cn=1/21+1/10+1/12
1/Cn=97/420
Cn=4.33F
Factors that affect Capacitance
- AREA OF PLATES
Capacitance is directly proportional to the plate area. The larger the plate area, the greater the capacitance, and the smaller the plate the less the capacitance.
- DIELECTRIC MATERIAL
All other factors being equal, the greater the dielectric constant(K) the greater the capacitance, and the less the dielectric constant(K) the less the capacitance.
| Material | Dielectric constant |
|---|---|
| Air or vacuum | 1 |
| Paper | 2.0-6.0 |
| Plastic | 2.1-6.0 |
| Mineral Oil | 2.2-2.3 |
| Polystyrene | 2.6 |
| Silicon Oil | 2.7-2.8 |
| Quartz | 3.8-4.4 |
| Glass | 4.8-8.0 |
| Porcelain | 5.1-5.9 |
| Mica | 5.4-8.7 |
| Askarel Oil | 5.6-5.9 |
| Aluminium Oxide | 8.4 |
| Tantalum Pentoxide | 26 |
| Ceramics | 12-400,000 |
- PLATE SEPARATION DISTANCE
All other factors being equal, the greater the distance the less the capacitance, and the less the distance the greater the capacitance. This is because capacitance is directly proportional to the electrostatic force field between the plates and the field is stronger when the plates are closer together.
In summary, capacitance is affected by:
- Area of plates
- Separation distance
- Dielectric material
FORMULA:
C=εA/d
where
C=capacitance in farads
A=Area of plate overlap
ε=permittivity of dielectric
D=distance between plates in meters

(Image source:slideplayer.com)
Example
A capacitor is constructed from two conductive metal plates 30cm x 50cm which are spaced 6mm apart from each other and uses dry air as its only dielectric material. Calculate the capacitance of the capacitor.(ε=8.854x10-12)
C=εA/d
A=0.3 x 0.5 m2
d=6 x10-3m
C=8.854x10-12X(0.3x0.5)/(6x10-3)
=0.221nF
REFERENCES
- Factors affecting capacitance| Dielectric constant. Electric Academia. https://electricalacademia.com/basic-electrical/factors-affecting-capacitance/
- Fowler (2003). Electricity Principles and Applications. SlidePlayer. https://slideplayer.com/slide/15391692/
- Factors affecting capacitance. All about circuits. https://www.allaboutcircuits.com/textbook/direct-current/chpt-13/factors-affecting-capacitance/
- Introduction to capacitors. Electronics tutorials. https://www.electronics-tutorials.ws/capacitor/cap_1.html
- Capacitance(PDF). http://www.phys.ufl.edu/courses/phy2054/s09/lectures/2054_ch16B.pdf
- Capacitors formulas. Electronics notes. https://www.electronics-notes.com/articles/basic_concepts/capacitance/capacitor-formulas-equations.php
Equations and Data Items
- Comments
- Attachments
- Stats
No comments |

