Tags | |
The Van't Hoff equation, ln(K2/K1) = ΔH0/R (1/T1 - 1/T2), provides information about the temperature dependence of the equilibrium constant. T
INSTRUCTIONS: Choose units and enter the following:
- (K1) Equilibrium constant at absolute temperature T1.
- (K2) Equilibrium constant at absolute temperature T2.
- (T1) Absolute temperature one.
- (T2 ) Absolute temperature two.
ΔH0: The calculator returns the reaction enthalpy (the standard heat of the reaction). This calculator uses the Ideal Gas Constant (R):8.3144626181532 J/(mol·K).
All temperatures are automatically converted to Kelvin.
The Math/Science
The Van't Hoff equation was proposed in 1884 by Jacobus Henricus van't Hoff. Van't Hoff made great contributions to physical chemistry, specifically in chemical kinetics, stereochemistry, and chemical equilibrium.
The formula in this equation is as follows:
`ΔH^0 = R * ( -ln(K_2/K_1))/ (1/T_1 - 1/T_2)`
It is a form of : ln(K2/K1) = ΔH0/R (1/T1 - 1/T2) solved for ΔH0.
If equilibrium constants are known for two different temperatures, then ΔH0 can be found for a substance. Likewise, if an equilibrium constant (K1) for T1 and ΔH0 are known, then the equilibrium constant (K2) can be calculated for a second temperature. The Van't Hoff equation does, however, assume that ΔH0 does not change with temperature. This is not necessarily true; ΔH0 does change with temperature, but the change is small enough that it is considered negligible when estimating K2.
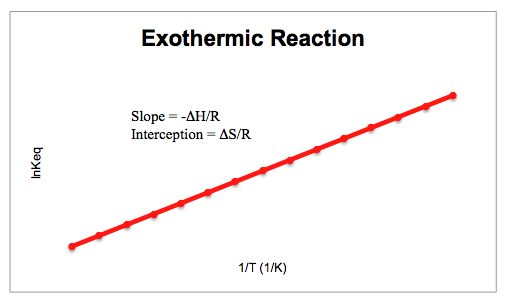
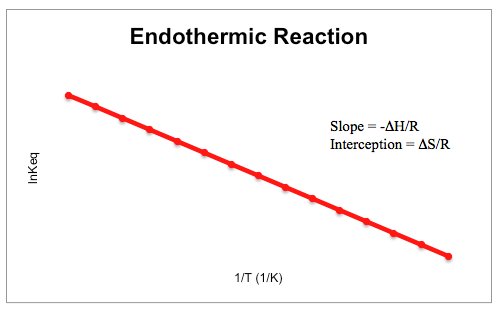
When the equilibrium constant and temperature are plotted against each other, both an exothermic and endothermic reaction will have a characteristic shape to its graph. Exothermic graphs tend to be linear with a positive slope, while endothermic reactions are linear with a negative slope, as shown below.
Chemistry Calculators
- R - Gas Constant: 8.3144626181532 J/(K⋅mol)
- Boyle's Law Calculator: P1 • V1 = P2 • V2
- Charles Law Calculator: V1• T2 = V2 • T1
- Combined Gas Law Calculator: P•V / T= k
- Gay-Lussac Law: T1•P2 =T2•P1
- Ideal Gas Law: P•V = n•R•T
- Bragg's Law: n·λ = 2d·sinθ
- Hess' Law: ΔH0rxn=ΔH0a+ΔH0b+ΔH0c+ΔH0d
- Internal Energy: ΔU = q + ω
- Activation Energy: Ea = (R*T1⋅T2)/(T1 - T2) ⋅ ln(k1/k2)
- Arrhenius Equation: k = AeE_a/(RT)
- Clausius-Clapeyron Equation: ln(P2/P1) = (ΔHvap)/R * (1/T1 - 1/T2)
- Compressibility Factor: Z = (p*Vm)/(R*T)
- Peng-Robinson Equation of State: p = (R*T)/(Vm - b) - (a*α)/(Vm2 + 2*b*Vm - b2)
- Reduced Specific Volume: vr = v/(R* Tcr / Pc)
- Van't Hoff Equation: ΔH0 = R * ( -ln(K2/K1))/ (1/T1 - 1/T2)
References
Whitten, et al. "Chemistry" 10th Edition. Pp. 699

