Bell-shaped Height

The Bell-shaped Height calculator computes the height associated with a bell-shaped object.

INSTRUCTIONS: Choose units and enter the following:

• (W) Width of Bell-shape

Bell-shaped Height (H): The calculator returns the height in meters.  However, this can be automatically converted to compatible units via the pull-down menu.

The Math / Science 

A bell-shaped area can be create by the intersection of two equal but reversed circle sectors (pieces of a pie) where the angle of the circle sector is 60°.  This creates an interior equilateral triangle (see diagram).

The formula for the height of a bell-shaped object is:

h = tan(π/3) ⋅ (W/2)

where:

• h = height of bell-shaped object
• W = base width of bell-shaped object
• π/3 = 60°

Bell-shaped Objects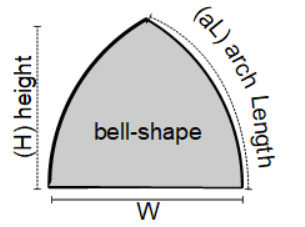

• Area of Bell-shaped Object
• Height of Bell-shaped Object
• Arc Length of Bell-shaped Object
• Volume of Bell-shaped Greenhouse

A bell-shaped area can be create by the intersection of two equal but reversed circle sectors (pieces of a pie) where the angle of the circle sector is 60° (π/3 radians).  This creates an interior equilateral triangle (see diagram).