30.1 A real image formed by a converging mirror by Benjamin Crowell, Light and Matter licensed under the Creative Commons Attribution-ShareAlike license.
30.1 A real image formed by a converging mirror
Location of the image
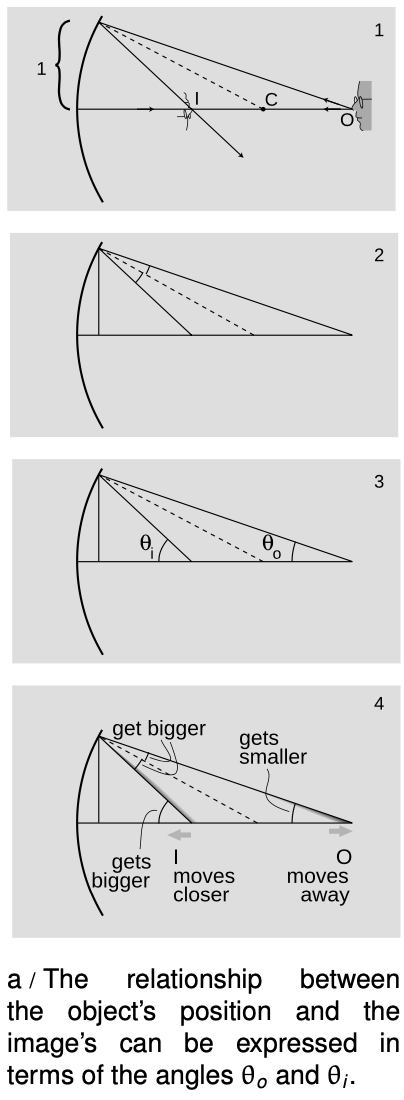We will now derive the equation for the location of a real image formed by a converging mirror. We assume for simplicity that the mirror is spherical, but actually this isn't a restrictive assumption, because any shallow, symmetric curve can be approximated by a sphere. The shape of the mirror can be specified by giving the location of its center, C. A deeply curved mirror is a sphere with a small radius, so C is close to it, while a weakly curved mirror has C farther away. Given the point O where the object is, we wish to find the point I where the image will be formed.
To locate an image, we need to track a minimum of two rays coming from the same point. Since we have proved in the previous chapter that this type of image is not distorted, we can use an on-axis point, O, on the object, as in figure a/1. The results we derive will also hold for off-axis points, since otherwise the image would have to be distorted, which we know is not true. We let one of the rays be the one that is emitted along the axis; this ray is especially easy to trace, because it bounces straight back along the axis again.
As our second ray, we choose one that strikes the mirror at a distance of 1 from the axis. “One what?” asks the astute reader. The answer is that it doesn't really matter. When a mirror has shallow curvature, all the reflected rays hit the same point, so 1 could be expressed in any units you like. It could, for instance, be 1 cm, unless your mirror is smaller than 1 cm!
The only way to find out anything mathematical about the rays is to use the sole mathematical fact we possess concerning specular reflection: the incident and reflected rays form equal angles with respect to the normal, which is shown as a dashed line. Therefore the two angles shown in figure a/2 are the same, and skipping some straightforward geometry, this leads to the visually reasonable result that the two angles in figure a/3 are related as follows:
`theta_i+theta_o="constant"`
(Note that `theta_i` and `theta_o`, which are measured from the image and the object, not from the eye like the angles we referred to in discussing angular magnification on page 838.) For example, move O farther from the mirror. The top angle in figure a/2 is increased, so the bottom angle must increase by the same amount, causing the image point, I, to move closer to the mirror. In terms of the angles shown in figure a/3, the more distant object has resulted in a smaller angle `theta_o`, while the closer image corresponds to a larger `theta_i`; One angle increases by the same amount that the other decreases, so their sum remains constant. These changes are summarized in figure a/4.
The sum `theta_i+theta_o` is a constant. What does this constant represent? Geometrically, we interpret it as double the angle made by the dashed radius line. Optically, it is a measure of the strength of the mirror, i.e., how strongly the mirror focuses light, and so we call it the focal angle, `theta_f`,
Suppose, for example, that we wish to use a quick and dirty optical test to determine how strong a particular mirror is. We can lay it on the floor as shown in figure c, and use it to make an image of a lamp mounted on the ceiling overhead, which we assume is very far away compared to the radius of curvature of the mirror, so that the mirror intercepts only a very narrow cone of rays from the lamp. This cone is so narrow that its rays are nearly parallel, and `theta_o` is nearly zero. The real image can be observed on a piece of paper. By moving the paper nearer and farther, we can bring the image into focus, at which point we know the paper is located at the image point. Since `theta_oapprox0`, we have `theta_iapproxtheta_f`, and we can then determine this mirror's focal angle either by measuring `theta_i` directly with a protractor, or indirectly via trigonometry. A strong mirror will bring the rays together to form an image close to the mirror, and these rays will form a blunt-angled cone with a large `theta_i` and `theta_f`.
Example 1: An alternative optical test
`=>` Figure c shows an alternative optical test. Rather than placing the object at infinity as in figure b, we adjust it so that the image is right on top of the object. Points O and I coincide, and the rays are reflected right back on top of themselves. If we measure the angle `theta` shown in figure c, how can we find the focal angle?
`=>` The object and image angles are the same; the angle labeled `theta` in the figure equals both of them. We therefore have `theta_i+theta_o=theta_a=theta_f`. Comparing figures b and c, it is indeed plausible that the angles are related by a factor of two.
At this point, we could consider our work to be done. Typically, we know the strength of the mirror, and we want to find the image location for a given object location. Given the mirror's focal angle and the object location, we can determine `theta_o` by trigonometry, subtract to find `theta_i=theta_f-theta_o`, and then do more trig to find the image location.
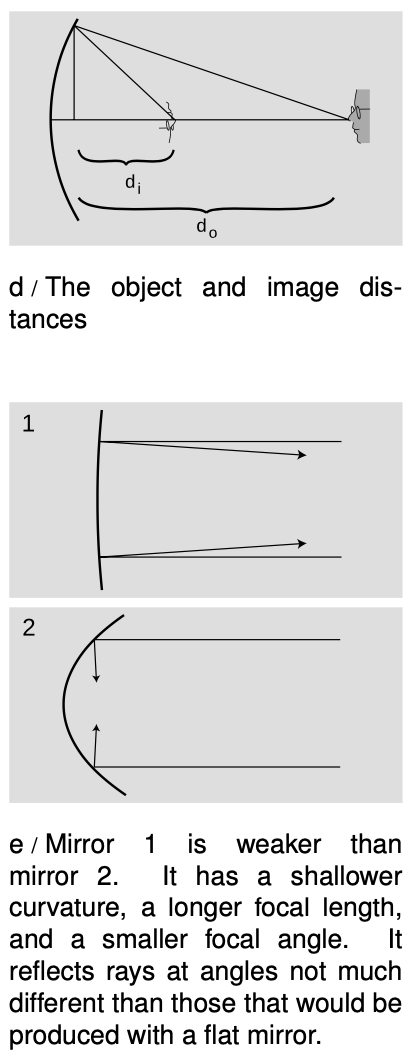
There is, however, a shortcut that can save us from doing so much work. Figure a/3 shows two right triangles whose legs of length 1 coincide and whose acute angles are `theta_o` and `theta_i`. These can be related by trigonometry to the object and image distances shown in figure d:
`tantheta_o=1"/"d_o`
`tantheta_i=1"/"d_i`
Ever since chapter 2, we've been assuming small angles. For small angles, we can use the small-angle approximation `tanxapproxx` (for `x` in radians), giving simply
`theta_o=1/d_o`
`theta_i=1/d_i`
We likewise define a distance called the focal length, `theta_f=1"/"f`. In figure b, `f` is the distance from the mirror to the place where the rays cross. We can now reexpress the equation relating the object and image positions as
`1/f=1/d_i+1/d_o`.
Figure e summarizes the interpretation of the focal length and focal angle.1
Which form is better, `theta_f=theta_i+theta_o` or `1"/"f=1"/"d_i+1"/"d_o`? The angular form has in its favor its simplicity and its straightforward visual interpretation, but there are two reasons why we might prefer the second version. First, the numerical values of the angles depend on what we mean by “one unit” for the distance shown as 1 in figure a/1. Second, it is usually easier to measure distances rather than angles, so the distance form is more convenient for number crunching. Neither form is superior overall, and we will often need to use both to solve any given problem.2
Example 2: A searchlight
Suppose we need to create a parallel beam of light, as in a searchlight. Where should we place the lightbulb? A parallel beam has zero angle between its rays, so `theta_i=0`. To place the lightbulb correctly, however, we need to know a distance, not an angle: the distance `d_o` between the bulb and the mirror. The problem involves a mixture of distances and angles, so we need to get everything in terms of one or the other in order to solve it. Since the goal is to find a distance, let's figure out the image distance corresponding to the given angle `theta_i=0`. These are related by `d_i=1/theta_i`, so we have `d_i=infty`. (Yes, dividing by zero gives infinity. Don't be afraid of infinity. Infinity is a useful problem-solving device.) Solving the distance equation for `d_o`, we have
`d_o=(1/f-1/d_i)^(-1)`
`=(1"/"f-0)^(-1)`
`=f`
The bulb has to be placed at a distance from the mirror equal to its focal point.
Example 3: Diopters
An equation like `d_i=1/theta_i` really doesn't make sense in terms of units. Angles are unitless, since radians aren't really units, so the right-hand side is unitless. We can't have a left-hand side with units of distance if the right-hand side of the same equation is unitless. This is an artifact of my cavalier statement that the conical bundles of rays spread out to a distance of 1 from the axis where they strike the mirror, without specifying the units used to measure this 1. In real life, optometrists define the thing we're calling `theta_i=1/d_i` as the “dioptric strength” of a lens or mirror, and measure it in units of inverse meters (`m^(-1)`), also known as diopters (`1 D=1 m^(-1)`).
Magnification
We have already discussed in the previous chapter how to find the magnification of a virtual image made by a curved mirror. The result is the same for a real image, and we omit the proof, which is very similar. In our new notation, the result is `M=d_i/d_o`. A numerical example is given in section 30.2.
30.1 A real image formed by a converging mirror by Benjamin Crowell, Light and Matter licensed under the Creative Commons Attribution-ShareAlike license.