Elevation of Object (angles and separation)

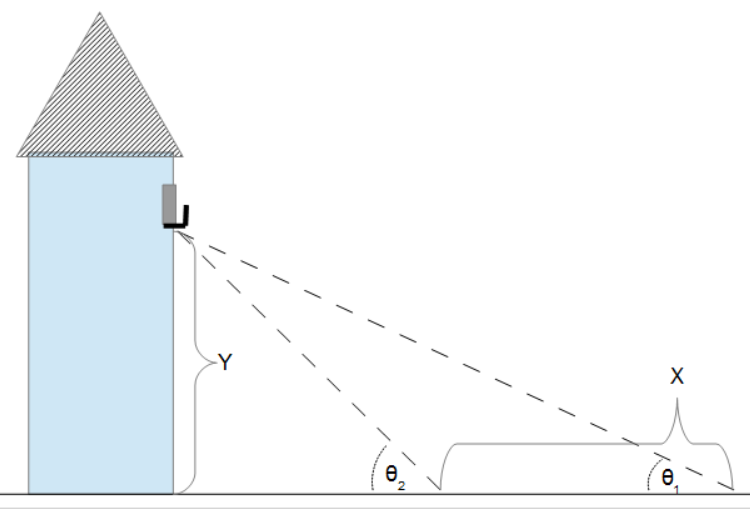
The Elevation of Object calculator computes the height of an object (Y) based on two angle measurements (θ₁ and θ₂) and the distance between them (x).

INSTRUCTIONS: Choose units and enter the following:

• (θ₁)  Angle from Further Observation
• (θ₂)  Angle from Nearer Observation
• (x)  Distance between Observations

Height of the Elevation(Y): The calculator returns the height in meters.  However, this can be automatically converted to other length units (e.g. feet) via the pull-down menu.

The Math / Science

The formula for the elevation or height of an object is:

        y = x /(cot(θ1) - cot(θ2))

where:

• y is the height or change in elevation of the object.
• x is the horizontal distance between observations.
• θ1 is the elevation angle from the further observation.
• θ2 is the elevation angle from the nearer observation.

Note: the assumption is that both observations are at the same elevation.  For a simple video on how this calculator is used, CLICK HERE.  For another method in computing the height of an elevation based the time an object falls from that height CLICK HERE.

Directions

This formula can be used to accurately compute the height of an object (Y) above the horizontal line made by two observations.   To do so, make one angle observation (`theta_1`) from any distance from the object.  Then, staying at approximately the same height, move closer to the object by a measured distance (x) and make a second angle observation (`theta_2`).  This formula will then compute the height (Y).

Applications

This formula is commonly used in science of surveys.  It is also useful in some architecture applications and in other activities such as Search and Rescue, Fire Fighting and even Rock Climbing.

Free Fall Calculators

• Free Fall (time): Computes the duration of time that an object will be in free fall based on the height and the acceleration due to gravity.
• Free Fall (distance): Computes the distance that an object will be in free fall based on the duration of the fall and the acceleration due to gravity.
• Free Fall (Velocity at Impact): Computes the final velocity of an object after a free fall based on the height and the acceleration due to gravity.
• Free Fall (Energy on Impact): Computes the kinetic energy at impact based on the height of free fall, acceleration due to gravity and the mass of the object.
• Model Rocket Altitude: Computes the estimated maximum altitude of a rocket based the distance from the launch point and the angle to top point of flight (zenith).
• Object Height by Time to Drop: Estimates the height of an object based on the time it takes for an object to drop from the top of it.
• Elevation of Object (angles and separation): Computes the height of an object based on two angle measurements and the distance between them.
• Distance Traveled at Constant Acceleration: Computes the distance traveled by an object after a period of time, based on its initial distance from the origin, initial velocity and a constant acceleration.
• g (acceleration due to gravity at sea level): Acceleration due to Gravity (g) at sea level on Earth is 9.80665 m/s².