Quantcast
Typesetting math: 100%

Bottle - mass

Last modified by
on
Mar 27, 2024, 5:43:44 PM
Created by
on
Aug 21, 2014, 2:56:02 PM
M=mD⋅[13⋅π⋅h2(a2+a⋅b+b2)+π⋅b2⋅h1]
(a)neck upper radius
(h2)tapered neck height
(b)body radius
(h1)body height
(mD)mean density
Tags
Geometry Mathematics Mass Euclidean Geometry (3D) Weight 3D bottle
UUID
4530c1d2-2943-11e4-b7aa-bc764e2038f2

The Mass of a Bottle calculator computes the mass based on the dimensions of the bottle and the mean density of the material. 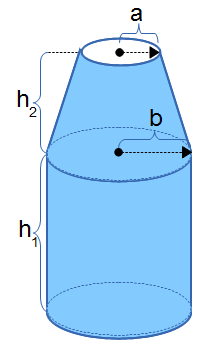Bottlevolume-illustration.png

 

INSTRUCTIONS: Choose the length units you wish (e.g. centimeters or inches) and enter the following: 

  • (a) neck radius at the top of the bottle
  • (h2) height of the tapered neck section
  • (b) base section's radius
  • (h1) height of the bottle's base that is a constant diameter along it's length from the bottom base to the beginning of the taper.
  • (mD) mean density of material.

Mass of the Bottle (M):  The calculator returns the mass in grams.  However, this can be automatically converted to compatible units via the pull-down menu.

Bottle Calculators

The Math / Science

The formula for the mass of a bottle shaped object is the volume of the bottle times the density of the material.  Assuming normal gravity, this also provides the weight.  Geometrically, a bottle shaped object is the combination of right circular cone with a frustum defined by base radius (a) and top radius (b) and height (h2) in between and a cylinder of radius (b) and height (h1).  The volume is calculated and then multiplied by a mean density (mD) to provide the mass of the shape.  See diagram.  KEY WORDS: container

Common Mean Densities

Natural

  • Pure Water - 1,000 kg/m³ 
  • Seawater - 1,022 kg/m³ 

Food

  • Milk - 1,037 kg/m³ 
  • Olive Oil - 860 kg/m³
  • Castor Oil - 961 kg/m3
  • Coconut Oil - 925 kg/m3
  • Soybean Oil - 926 kg/m3
  • Sunflower Oil - 920 kg/m3

Industrial

  • Cement Slurry - 1,442 kg/m³
  • Cotton seed Oil - 925.87 kg/m3
  • Linseed Oil - 929.07 kg/m3
  • Mercury - 13,534 kg/m3
  • Sewage Sludge - 721 kg/m3

Fuels

  • Diesel Fuel - 885 kg/m³ 
  • Crude Oil - 870 kg/m³  to 920 kg/m³ 
  • Fuel Oil -  890 kg/m³ 
  • Ethanol - 789 kg/m³ 
  • Gasoline (petrol) - 737 kg/m³ 
  • Kerosene - 817.15 kg/m3
  • Propane - 493 kg/m3
  • Liquid Natural Gas - 430 to 470 kg/m3

Mean Density of Fluids 

Mean density is the average amount of mass within a volume for a substance.  Note, volume of a material is often highly subject to the temperatures, since materials expand as they warm.  For that reason, mean densities of substances are often cited with a set of nominal conditions such as temperature and barometric pressure. 

The formula for mean density is:

μD = V / m

where:

  • μD = mean density
  • V = Volume in units like gallons or liters
  • m = Mass in units like kilograms or pounds

Mean density is also often indicated as the Greek symbol rho (ρ).

The mean density of fluids can be useful since fluids (liquids and gases) conform to the shape of their containers.  This is why it is possible to use the mean density of a substance and the dimensions of its container to estimate the weight/mass of the substance in the container.

 

This equation, Bottle - mass, is used in 4 pages
  • Comments
  • Attachments
  • Stats
No comments
This site uses cookies to give you the best, most relevant experience. By continuing to browse the site you are agreeing to our use of cookies.