Ballistic Position (X,Y)
Tags | |
UUID | 08be8e7f-94c3-11e8-abb7-bc764e2038f2 |
The Ballistic Position calculator computes the vertical (y component) and horizontal (x) position based on an initial velocity, launch angle, and time.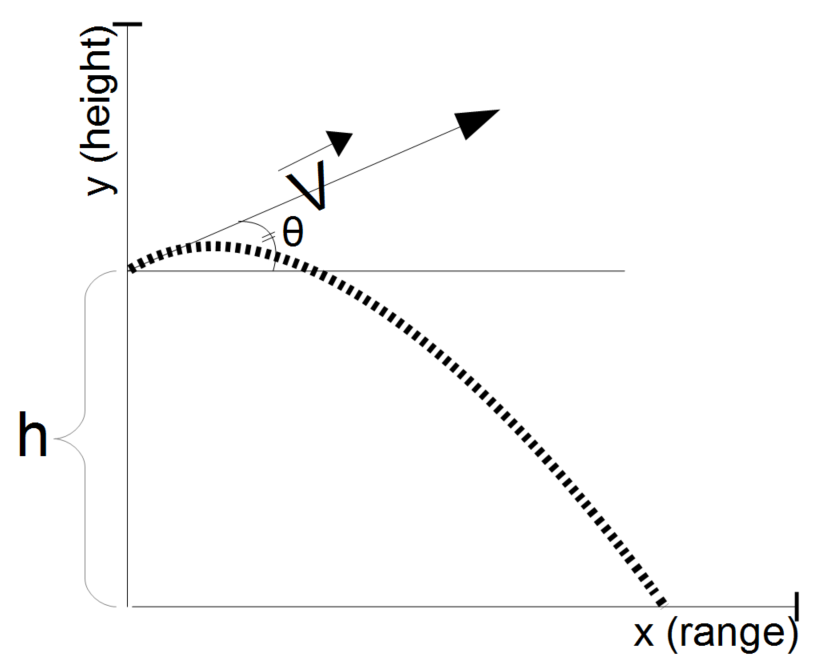
INSTRUCTIONS: Choose units and enter the following:
- (V) Magnitude of Initial Velocity
- (Θ) Launch Angle
- (t) Time into Ballistic Flight
- (h) Initial Height above the plane.
Position (x,y): The calculator returns the relative position in meters, where x is the distance down range and y is height above the plane.
The Math / Science
This calculator first computes the maximum flight time. If the time entered is greater than the max flight time, the time is set to the max flight time in order to compute the X, Y position. This implies that the object is at rest after the max flight time.
The Ballistic Vertical Position equation calculates an object's position in the y direction, the vertical component of its ballistic trajectory. This is the equation for the ideal projectile, which means it neglects external forces such as air resistance. Since in the ideal projectile motion model the force of gravity is the only force component affecting the trajectory, and the force of gravity is parallel to the y-component of motion, the gravitational acceleration is the only affect the ballistic y-velocity. The y-component of position changes based on the time and the constant acceleration. The formula for the vertical position is:
where:
- y is the vertical position
- V0 is the initial velocity
- θ is the launch angle
- t is the time into the flight
- h is the height above the plane
- g is the acceleration due to gravity
The Ballistic Horizontal Position equation calculates an object's position in the x direction, the horizontal component of its ballistic trajectory. This is the equation for the ideal projectile, which means it neglects external forces such as air resistance. Since in the ideal projectile motion model the force of gravity is the only force component affecting the trajectory, and the force of gravity is parallel to the y-component of motion, the gravitational acceleration has no affect the ballistic x-velocity. The x-component of position changes based on the time and the horizontal velocity. The formula for the horizontal position is:
where:
- x is the horizontal position
- V0 is the initial velocity
- θ is the launch angle
- t is the time into the flight

Ballistic Flight Calculators:
- Ballistic Maximum Altitude: This is the maximum altitude achieved in free ballistic flight.
- Ballistic Maximum Range: This is the maximum horizontal range.
- Ballistic Flight Time: This is the time duration of free flight.
- Simplified Ballistic Range: This is the range with no initial elevation above the plane.
- Ballistic Vertical Velocity: This is the vertical velocity at a given time.
- Ballistic Horizontal Velocity: This is horizontal velocity or ground speed.
- Vertical Position (Y) in Ballistic Flight: This compute the vertical position (y) at a given time within ballistic flight.
- Horizontal Position (X) in Ballistic Flight: This compute the horizontal position (x) at a given time within ballistic flight..
- Ballistic Position at Time (t): This compute the position (x,y) at a given time within ballistic flight, where x is distance down range and y is the height above the plane.
- Ballistic Parabolic Equation provides the parabolic flight position equation based on the launch speed, height and angle.
- Acceleration Due to Gravity at Sea Level
- Velocity to achieve a Max Ballistic Height: This computes the initial velocity required to achieve the max height.
- International Gravity Equation
- Force of Earth's Gravity
- Force of Drag
Equations and Data Items
Calculators
- Comments
- Attachments
- Stats
No comments |
