Vector Rotation

The Vector Rotation calculator computes the resulting 3D vector created by rotating a base vector (V) about a rotation vector (U) by an angle(α).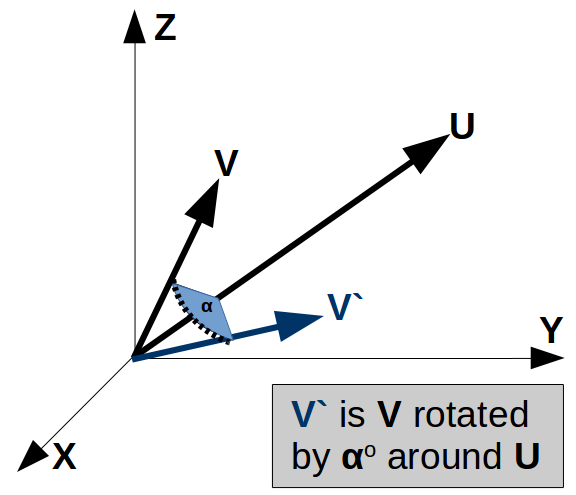

INSTRUCTIONS: Enter the following:

• (V):  Base vector (V) to be rotated
• (U):  Rotation axis vector (U) 
• (α):  Rotation angle
• (n):  Number of Significant Digits

Rotated Vector (V'): The calculator returns the resultant vector (V') 

The Math / Science

The Vector Rotation formula uses quaternions to compute the resulting vector from the specified rotation.  It uses the rotation of axis (U) and the rotation angle (α) to compute the quaternion of rotation (q).  It then uses the quaternion vector rotation formula as follows:

           V' = q⋅V⋅q^*
where:

• V' is the rotated resultant vector.
• q is the quaternion of rotation
• q^* is conjugate of the quaternion of rotation.

3D Vector Functions

• k⋅V - scalar multiplication
• V/k - scalar division
• V / |V| - Computes the Unit Vector
• |V| - Computes the magnitude of a vector
• U + V - Vector addition
• U - V - Vector subtraction
• |U - V| - Distance between vector endpoints.
• |U + V| - Magnitude of vector sum.
• V • U - Computes the dot product of two vectors
• V x U - Computes the cross product of two vectors
• V x U • W - Computes the mixed product of three vectors
• Vector Angle - Computes the angle between two vectors
• Vector Area - Computes the area between two vectors
• Vector Projection - Compute the vector projection of V onto U.
• Vector Rotation - Compute the result vector after rotating around an axis.
• Vector Components 3D - Returns a vector's magnitude, unit vector, spherical coordinates, cylindrical coordinates and angle from each axis.
• (ρ, θ, φ) to (x,y,z) - Spherical to Cartesian coordinates
• (x,y,z) to (ρ, θ, φ) - Cartesian to Spherical coordinates
• (r, θ, z) to (x,y,z) - Cylindrical to Cartesian coordinates
• (x,y,z) to (r, θ, z) - Cartesian to Cylindrical coordinates
• (x,y) to (r, θ) - Cartesian to Polar
• (r, θ) to (x,y) - Polar to Cartesian 
• Vector Normal to a Plane Defined by Three Points

Quaternion Calculators

The Quaternion Calculator includes functions associated with quaternion mathematics. The quaternions are in the form of "scalar first" (q4,q1,q2,q3).  The quaternion arithmetic functions include the following:

• Quaternion Addition
• Quaternion Subtraction
• Quaternion Multiplication
• Quaternion Dot Product
• Quaternion Magnitude
• Quaternion VersorQUATERNION ROTATIONS
• Quaternion Conjugate
• Quaternion Inverse
• Quaternion of Rotation
• Vector Rotation

Quaternions

Quaternions can be represented in several ways. One of the ways is similar to the way complex numbers are represented:
               q ≡ q₄ + q₁i + q₂j + q₃k,

in which q₁ , q₂ , q₃ and q₄ , are real numbers, and i, j, and k, are unit “vectors” which obey similar rules to the vectors of the same names found in vector analysis, but with an additional similarity to the i of complex arithmetic which equals `sqrt(-1)` . The multiplication rules for i , j , and k are depicted
conceptually as follows: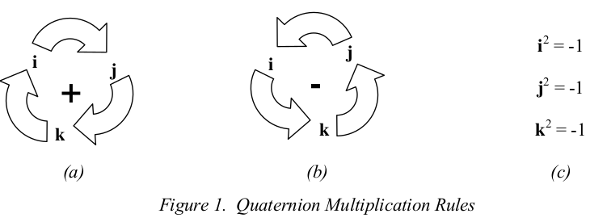

That is, i j = + k, j k = + i, etc. , from figure 1(a) , and j i = - k, i k = -j , etc., from figure 1(b) . Expressed
in this form, the multiplication rules are very easy to remember. Note that the cross products of i, j , and
k obey the rules of vector cross product multiplication, where, for example, given the orthogonal axes,
x, y, and z: x × y = z, y × z = x , and z × x = y .

Note: Quaternions are not commutative, and the following should be noted:

             q₁*q₂ ≠ q₂ * q₁
                   q₁*q₂ ≠ -q₂ * q₁, but
      (q₁ * q₂) * q₃ = q₁ * (q₂ * q₃)

Use of Quaternions in Aerospace

Quaternions are very good for defining the rotation between two coordinate frames.  For this reason, time tagged quaternions are used to indicate the attitude (orientation) of a satellite at a specific moment.  With an attitude quaternion, satellite mission planners can determine the rotations it takes to go from one attitude to another.  This is how sensors on board of satellites are modeled and moved.  The same is true for other fixed components such as solar arrays and communication antennae. 

Vector Matrix and Quaternion Calculators

• 3D Vector Calculator
• Matrix Multiplication Calculator
• Quaternion Calculator

References

• Light and Matter by Benjamin Crowell, Chapter 7.1 Vector Notation
• An Engineer's Approach to Quaternions* (Damon D. Ostrander October 1998)  See Quaternions RevA.pdf (attached).